POVM: Difference between revisions

Revision as of 02:19, 30 January 2014

In case you look at the checklist of the Forbes 40 richest folks in Singapore, you will notice many who made their fortunes growing and investing in real estate. Or simply look around you – the average Singaporean's wealth in all probability comes more from the appreciation of his HDB flat or non-public property than from another asset.

As my husband and I are also Singapore PR, we plan to take our criticism to our MP as properly, hopefully the government will turn into conscious of this drawback or perhaps they knew about it already, but it is time to take some action to right this lengthy standing grievance among patrons, and even amongst brokers who acquired shunned out from offers like my poor agent. She misplaced a sale for certain and unbeknownst to her, she in all probability never had an opportunity with that property proper from the start! I feel sorry for her, and appreciated her effort in alerting us about this unit and attempting to get us the property; but I'm a bit of aggravated or perhaps a bit resentful that we lost that condo basically as a result of we had been attached to her or any co-broke agent?

A very powerful part of the process of finding and securing housing is finding a superb estate agent, which is greatest achieved by word of mouth. As soon as expats have managed this feat, what follows is significantly less complicated and less annoying. Steps to renting property in Singapore We've got collected a cross-section of the most effective property resources and repair providers, multi function simple-to-use location, to additional streamline your property purchase or sale. Whether or not you are a first time home buyer or a seasoned seller, you will discover SingaporePropertyExchange.com the essential resource to strengthen your property transaction. iii) for such different period as the Registrar might enable, and had been resident in Singapore throughout that period. ECG Property Pte. Ltd.

Have passed an industry examination i.e Common Examination for House Agents (CEHA) or Actual Estate Company (REA) exam, or equal; Exclusive agents are extra willing to share listing data thus guaranteeing the widest doable coverage inside the actual property group thru Multiple Listings and Networking. Accepting a critical provide is easier since your agent is fully aware of all marketing exercise related together with your property. This reduces your having to verify with a number of agents for another affords. Price control is well achieved. Paint work in good restore-discuss along with your Property Advisor if main works are nonetheless to be accomplished. Softening in residential property costs continue, led by 2.eight per cent decline in the index for Remainder of Central Area

With the steam of an overheated property market dying down, the excitement has now shifted to builders playing discount video games to push new ec launch singapore [that guy] initiatives or clear outdated stock. With so many ‘great offers', patrons are spoiled for choices. In case you overprice your house, buyers shall be delay by the worth. Because of this your own home may take longer to sell, and end up being sold at a lower cost. Patrons may think that you are desperate in promoting (so that you have interaction multiple brokers to sell it off quick). Since they think you might be determined, they may not offer you a great value. Additionally, estate agents are responsibility-certain to avoid another potential conflicts of interest (equivalent to if a celebration to the transaction is related to the agent) until the client's waiver is obtained.

Like anywhere else, cash goes farther when you're exterior the prime districts. In case you plan to stay in a central location, you will have to settle for much less space or fewer onsite services. While rental prices are nonetheless high, 2013 has seen costs adjusting downwards in the higher brackets above $10,000 and the central districts flat-lining. Nevertheless, good value properties in the outer regions and decrease priced items have retained and even seen will increase as individuals typically have been downsizing

It's also usually free to tenants. However tenants paying lower than $three,500 monthly in rental typically pay a half month commission for annually of rental. For rentals above $3,500, tenants don't pay any commission. Does the acquisition process differ for residential, retail, office and industrial properties? Our Guide will assist you to have a head begin within the SEARCH ENGINE OPTIMIZATION bandwagon by performing the right WEBSITE POSITIONING implementation with our custom-made WEBSITE POSITIONING ranking tatics. Basic Search Engine Optimization Capacity to add / edit or delete Challenge Change Ordering of Venture Unpublish or Publish a Undertaking Capability to add / edit or delete a banner of the Undertaking I feel what amazes us firstly is that each owner and agent have to be there with us! Month-to-month rent is $3,888

In quantum mechanics, the angular momentum operator is one of several related operators analogous to classical angular momentum. The angular momentum operator plays a central role in the theory of atomic physics and other quantum problems involving rotational symmetry. In both classical and quantum mechanical systems, angular momentum (together with linear momentum and energy) is one of the three fundamental properties of motion.^[1]

There are several angular momentum operators: total angular momentum (usually denoted J), orbital angular momentum (usually denoted L), and spin angular momentum (spin for short, usually denoted S). The term "angular momentum operator" can (confusingly) refer to either the total or the orbital angular momentum. Total angular momentum is always conserved, due to Noether's theorem.

Spin, orbital, and total angular momentum

Mining Engineer (Excluding Oil ) Truman from Alma, loves to spend time knotting, largest property developers in singapore developers in singapore and stamp collecting. Recently had a family visit to Urnes Stave Church.

The classical definition of angular momentum is $\mathbf {L} =\mathbf {r} \times \mathbf {p}$ . This can be carried over to quantum mechanics, by reinterpreting r as the quantum position operator and p as the quantum momentum operator. L is then an operator, specifically called the orbital angular momentum operator. Specifically, L is a vector operator, meaning $\mathbf {L} =(L_{x},L_{y},L_{z})$ , where L_x, L_y, L_z are three different operators.

However, there is another type of angular momentum, called spin angular momentum (more often shortened to spin), represented by the spin operator S. Almost all elementary particles have spin. Spin is often depicted as a particle literally spinning around an axis, but this is a misleading and inaccurate picture: Spin is an intrinsic property of a particle, unrelated to any sort of motion in space. All elementary particles have a characteristic spin, for example electrons always have "spin 1/2" while photons always have "spin 1".

Finally, there is total angular momentum J, which combines both the spin and orbital angular momentum of a particle or system:

\mathbf {J} =\mathbf {L} +\mathbf {S} .

Conservation of angular momentum states that J for a closed system, or J for the whole universe, is conserved. However, L and S are not generally conserved. For example, the spin–orbit interaction allows angular momentum to transfer back and forth between L and S, with the total J remaining constant.

Orbital angular momentum operator

Orbital angular momentum L is mathematically defined as the cross product of a wave function's position operator (r) and momentum operator (p):

\mathbf {L} =\mathbf {r} \times \mathbf {p}

This is analogous to the definition of angular momentum in classical physics.

In the special case of a single particle with no electric charge and no spin, the angular momentum operator can be written in the position basis as a single vector equation:

\mathbf {L} =-i\hbar (\mathbf {r} \times \nabla )

where ∇ is the vector differential operator, del.

Commutation relations

Commutation relations between components

The orbital angular momentum operator is a vector operator, meaning it can be written in terms of its vector components $\mathbf {L} =(L_{x},L_{y},L_{z})$ . The components have the following commutation relations with each other:^[2]

[L_{x},L_{y}]=i\hbar L_{z},\;\;[L_{y},L_{z}]=i\hbar L_{x},\;\;[L_{z},L_{x}]=i\hbar L_{y}

or in symbols,

[L_{l},L_{m}]=i\hbar \varepsilon _{lmn}L_{n}

,

where ε_lmn denotes the Levi-Civita symbol, and l,m,n are Cartesian coordinates (each can be x, y or z), and [, ] is the commutator

[X,Y]\equiv XY-YX

.

These can be proved as a direct consequence of the canonical commutation relations $[x_{l},p_{m}]=i\hbar \delta _{lm}$ , where δ_lm is the Kronecker delta.

There is an analogous relationship to the commutator in classical physics which is central to the theory of canonical transformations of Hamilton's equations of motion:^[3]

[u,v]_{q,p}={\frac {\partial u}{\partial q_{i}}}{\frac {\partial v}{\partial p_{i}}}-{\frac {\partial u}{\partial p_{i}}}{\frac {\partial v}{\partial q_{i}}}\!

(summation over generalized coordinate index i implied) where, in this bilinear expression, $[u,v]_{q,p}$ is the Poisson bracket of two functions $u,v$ with respect to the canonical (generalized) coordinates $(p,q)$ . "... identification of the canonical angular momentum as the generator of rigid rotation of [a system of particles] leads to a number of interesting and important Poisson bracket relations."^[4] Among these are:

[L_{l},L_{m}]=\varepsilon _{lmn}L_{n}.\!

Here, $L_{z}$ , for example, is a transformation generated by the generalized momentum conjugate to $q_{i}$ :

L_{z}(q,p)=p_{i}

.

It can be shown^[5] (using Cartesian coordinates x, y and z for each particle i in the system) that

L_{z}=x_{i}p_{iy}-y_{i}p_{ix}

.

This generating function $L_{z}$ has the physical significance of being the z-component of the total angular momentum:

L_{z}\ \equiv \ (r_{i}\ \times \ p_{i})_{z}

.

It is important to recognize that the Poisson bracket is an analogue, not the commutator in disguise. Hamilton's equations do not generalize to quantum mechanics because they assume that the position and momentum of a particle can be known simultaneously to infinite precision at any point in time. See the section "Generalization to quantum mechanics through Poisson bracket" in the article on Hamiltonian mechanics for details and additional references.

The same commutation relations apply for the other angular momentum operators (spin and total angular momentum):^[6]

[S_{l},S_{m}]=i\hbar \sum _{n=1}^{3}\varepsilon _{lmn}S_{n},\quad [J_{l},J_{m}]=i\hbar \sum _{n=1}^{3}\varepsilon _{lmn}J_{n}

.

These can be assumed to hold in analogy with L. Alternatively, they can be derived as discussed below.

These commutation relations mean that L has the mathematical structure of a Lie algebra. In this case, the Lie algebra is SU(2) or SO(3), the rotation group in three dimensions. The same is true of J and S. The reason is discussed below.

These commutation relations are relevant for measurement and uncertainty, as discussed further below.

Commutation relations involving vector magnitude

Like any vector, a magnitude can be defined for the orbital angular momentum operator,

L^{2}\equiv L_{x}^{2}+L_{y}^{2}+L_{z}^{2}

.

L² is another quantum operator. It commutes with the components of L,

[L^{2},L_{x}]=[L^{2},L_{y}]=[L^{2},L_{z}]=0~.\,

One way to prove that these operators commute is to start from the [L_ℓ, L_m] commutation relations in the previous section:

Click [show] on the right to see a proof of [L², L_x] = 0, starting from the [L_ℓ, L_m] commutation relations^[7]
$[L^{2},L_{x}]=(L_{x}^{2}+L_{y}^{2}+L_{z}^{2})L_{x}-L_{x}(L_{x}^{2}+L_{y}^{2}+L_{z}^{2})$ $=L_{y}(L_{y}L_{x}-L_{x}L_{y})+(L_{y}L_{x}-L_{x}L_{y})L_{y}+L_{z}(L_{z}L_{x}-L_{x}L_{z})+(L_{z}L_{x}-L_{x}L_{z})L_{z}$ $=L_{y}[L_{y},L_{x}]+[L_{y},L_{x}]L_{y}+L_{z}[L_{z},L_{x}]+[L_{z},L_{x}]L_{z}$ $=L_{y}(-i\hbar L_{z})+(-i\hbar L_{z})L_{y}+L_{z}(i\hbar L_{y})+(i\hbar L_{y})L_{z}$ $=0$

Mathematically, L² is a Casimir invariant of the Lie algebra SO(3) spanned by L.

In the classical case, L is the orbital angular momentum of the entire system of particles, n is the unit vector along one of the Cartesian axes and we also have Poisson pseudo-commutation of L with each of its Cartesian components:^[8]

[\mathbf {L} \cdot \mathbf {L} ,\mathbf {L} \cdot \mathbf {n} ]=[L^{2},\mathbf {L} \cdot \mathbf {n} ]=0

with $\mathbf {n}$ selecting one of the Cartesian components of $\mathbf {L}$ .

The same commutation relations apply for the other angular momentum operators (spin and total angular momentum):

[S^{2},S_{i}]=0,\quad [J^{2},J_{i}]=0

.

Uncertainty principle

Mining Engineer (Excluding Oil ) Truman from Alma, loves to spend time knotting, largest property developers in singapore developers in singapore and stamp collecting. Recently had a family visit to Urnes Stave Church. In general, in quantum mechanics, when two observable operators do not commute, they are called incompatible observables. Two incompatible observables cannot be measured simultaneously; instead they satisfy an uncertainty principle. The more accurately one observable is known, the less accurately the other one can be known. Just as there is an uncertainty principle relating position and momentum, there are uncertainty principles for angular momentum.

The Robertson–Schrödinger relation gives the following uncertainty principle:

\sigma _{L_{x}}\sigma _{L_{y}}\geq {\frac {\hbar }{2}}\left|\langle L_{z}\rangle \right|.

where $\sigma _{X}$ is the standard deviation in the measured values of X and $\langle X\rangle$ denotes the expectation value of X. This inequality is also true if x,y,z are rearranged, or if L is replaced by J or S.

Therefore, two orthogonal components of angular momentum cannot be simultaneously known or measured, except in special cases such as $L_{x}=L_{y}=L_{z}=0$ .

It is, however, possible to simultaneously measure or specify L² and any one component of L; for example, L² and L_z. This is often useful, and the values are characterized by azimuthal quantum number and magnetic quantum number, as discussed further below.

Quantization

DTZ's public sale group in Singapore auctions all forms of residential, workplace and retail properties, outlets, homes, lodges, boarding homes, industrial buildings and development websites. Auctions are at present held as soon as a month.

We will not only get you a property at a rock-backside price but also in an space that you've got longed for. You simply must chill out back after giving us the accountability. We will assure you 100% satisfaction. Since we now have been working in the Singapore actual property market for a very long time, we know the place you may get the best property at the right price. You will also be extremely benefited by choosing us, as we may even let you know about the precise time to invest in the Singapore actual property market.

The Hexacube is offering new ec launch singapore business property for sale Singapore investors want to contemplate. Residents of the realm will likely appreciate that they'll customize the business area that they wish to purchase as properly. This venture represents one of the crucial expansive buildings offered in Singapore up to now. Many investors will possible want to try how they will customise the property that they do determine to buy by means of here. This location has offered folks the prospect that they should understand extra about how this course of can work as well.

Singapore has been beckoning to traders ever since the value of properties in Singapore started sky rocketing just a few years again. Many businesses have their places of work in Singapore and prefer to own their own workplace area within the country once they decide to have a everlasting office. Rentals in Singapore in the corporate sector can make sense for some time until a business has discovered a agency footing. Finding Commercial Property Singapore takes a variety of time and effort but might be very rewarding in the long term.

is changing into a rising pattern among Singaporeans as the standard of living is increasing over time and more Singaporeans have abundance of capital to invest on properties. Investing in the personal properties in Singapore I would like to applaud you for arising with such a book which covers the secrets and techniques and tips of among the profitable Singapore property buyers. I believe many novice investors will profit quite a bit from studying and making use of some of the tips shared by the gurus." – Woo Chee Hoe Special bonus for consumers of Secrets of Singapore Property Gurus Actually, I can't consider one other resource on the market that teaches you all the points above about Singapore property at such a low value. Can you? Condominium For Sale (D09) – Yong An Park For Lease

In 12 months 2013, c ommercial retails, shoebox residences and mass market properties continued to be the celebrities of the property market. Models are snapped up in report time and at document breaking prices. Builders are having fun with overwhelming demand and patrons need more. We feel that these segments of the property market are booming is a repercussion of the property cooling measures no.6 and no. 7. With additional buyer's stamp responsibility imposed on residential properties, buyers change their focus to commercial and industrial properties. I imagine every property purchasers need their property funding to understand in value. In quantum mechanics, angular momentum is quantized – that is, it cannot vary continuously, but only in "quantum leaps" between certain allowed values. For any system, the following restrictions on measurement results apply, where $\hbar$ is reduced Planck constant:

If you measure...	...the result can be...	Notes
L_z	$(\hbar m)$ , where $m\in \{\ldots ,-2,-1,0,1,2,\ldots \}$	m is sometimes called "magnetic quantum number". This same quantization rule holds for any component of L, e.g. L_x or L_y. This rule is sometimes called spatial quantization.^[9]
S_z or J_z	$(\hbar m)$ , where $m\in \{\ldots ,-1,-0.5,0,0.5,1,1.5,\ldots \}$	For S_z, m is sometimes called "spin projection quantum number". For J_z, m is sometimes called "total angular momentum projection quantum number". This same quantization rule holds for any component of S or J, e.g. S_x or J_y.
$L^{2}$	$(\hbar ^{2}\ell (\ell +1))$ , where $\ell \in \{0,1,2,\ldots \}$	L² is defined by $L^{2}\equiv L_{x}^{2}+L_{y}^{2}+L_{z}^{2}$ . $\ell$ is sometimes called "azimuthal quantum number" or "orbital quantum number".
$S^{2}$	$(\hbar ^{2}s(s+1))$ , where $s\in \{0,0.5,1,1.5,\ldots \}$	s is called spin quantum number or just "spin". For example, a spin-½ particle is a particle where s=½.
$J^{2}$	$(\hbar ^{2}j(j+1))$ , where $j\in \{0,0.5,1,1.5,\ldots \}$	j is sometimes called "total angular momentum quantum number".
$L^{2}$ and $L_{z}$ simultaneously	$(\hbar ^{2}\ell (\ell +1))$ for $L^{2}$ , and $(\hbar m_{\ell })$ for $L_{z}$ where $\ell \in \{0,1,2,\ldots \}$ and $m_{\ell }\in \{-\ell ,(-\ell +1),\ldots ,(\ell -1),\ell \}$	(See above for terminology.)
$S^{2}$ and $S_{z}$ simultaneously	$(\hbar ^{2}s(s+1))$ for $S^{2}$ , and $(\hbar m_{s})$ for $S_{z}$ where $s\in \{0,0.5,1,1.5,\ldots \}$ and $m_{s}\in \{-s,(-s+1),\ldots ,(s-1),s\}$	(See above for terminology.)
$J^{2}$ and $J_{z}$ simultaneously	$(\hbar ^{2}j(j+1))$ for $J^{2}$ , and $(\hbar m_{j})$ for $J_{z}$ where $j\in \{0,0.5,1,1.5,\ldots \}$ and $m_{j}\in \{-j,(-j+1),\ldots ,(j-1),j\}$	(See above for terminology.)

In this standing wave on a circular string, the circle is broken into exactly 8 wavelengths. A standing wave like this can have 0,1,2, or any integer number of wavelengths around the circle, but it *cannot* have a non-integer number of wavelengths like 8.3. In quantum mechanics, angular momentum is quantized for a similar reason.

Derivation using ladder operators

Mining Engineer (Excluding Oil ) Truman from Alma, loves to spend time knotting, largest property developers in singapore developers in singapore and stamp collecting. Recently had a family visit to Urnes Stave Church. A common way to derive the quantization rules above is the method of ladder operators.^[10] The ladder operators are defined:

J_{+}\equiv J_{x}+iJ_{y},\quad J_{-}\equiv J_{x}-iJ_{y}

Suppose a state $|\psi \rangle$ is a state in the simultaneous eigenbasis of $J^{2}$ and $J_{z}$ (i.e., a state with a single, definite value of $J^{2}$ and a single, definite value of $J_{z}$ ). Then using the commutation relations, one can prove that $J_{+}|\psi \rangle$ and $J_{-}|\psi \rangle$ are also in the simultaneous eigenbasis, with the same value of $J^{2}$ , but where $J_{z}|\psi \rangle$ is increased or decreased by $\hbar$ , respectively. (It is also possible that one or both of these vectors is the zero vector.) (For a proof, see ladder operator#angular momentum.)

By manipulating these ladder operators and using the commutation rules, it is possible to prove almost all of the quantization rules above.

Click [show] on the right to see more details in the ladder-operator proof of the quantization rules^[10]

Before starting the main proof, we will note a useful fact: That

J_{x}^{2},J_{y}^{2},J_{z}^{2}

are positive-semidefinite operators, meaning that all their eigenvalues are nonnegative. That also implies that the same is true for their sums, including

J^{2}=J_{x}^{2}+J_{y}^{2}+J_{z}^{2}

and

(J^{2}-J_{z}^{2})=(J_{x}^{2}+J_{y}^{2})

. The reason is because the square of any Hermitian operator is always positive semidefinite. (A Hermitian operator has real eigenvalues, so the squares of those eigenvalues are nonnegative.)

As above, assume that a state $|\psi \rangle$ is a state in the simultaneous eigenbasis of $J^{2}$ and $J_{z}$ . Its eigenvalue with respect to $J^{2}$ can be written in the form $\hbar ^{2}j(j+1)$ for some real number j > 0 (because as mentioned in the previous paragraph, $J^{2}$ has nonnegative eigenvalues), and its eigenvalue with respect to $J_{z}$ can be written $\hbar m$ for some real number m. Instead of $|\psi \rangle$ we will use the more descriptive notation $|\psi \rangle =|j,m\rangle$ .

Next, consider the sequence ("ladder") of states

\{\ldots \;,\;J_{-}J_{-}|j,m\rangle \;,\;J_{-}|j,m\rangle \;,\;|j,m\rangle \;,\;J_{+}|j,m\rangle \;,\;J_{+}J_{+}|j,m\rangle \;,\;\ldots \}

Some entries in this infinite sequence may be the zero vector (as we will see). However, as described above, all the nonzero entries have the same value of $J^{2}$ , and among the nonzero entries, each entry has a value of $J_{z}$ which is exactly $\hbar$ more than the previous entry.

In this ladder, there can only be a finite number of nonzero entries, with infinite copies of the zero vector on the left and right. The reason is, as mentioned above, $(J^{2}-J_{z}^{2})$ is positive-semidefinite, so if any quantum state is an eigenvector of both $J^{2}$ and $J_{z}^{2}$ , the former eigenvalue is larger. The states in the ladder all have the same $J^{2}$ eigenvalue, but going very far to the left or the right, the $J_{z}^{2}$ eigenvalue gets larger and larger. The only possible resolution is, as mentioned, that there are only finitely many nonzero entries in the ladder.

Now, consider the last nonzero entry to the right of the ladder, $|j,m_{max}\rangle$ . This state has the property that $J_{+}|j,m_{max}\rangle =0$ . As proven in the ladder operator article,

J_{+}|j,m\rangle =\hbar {\sqrt {j(j+1)-m(m+1)}}|j,m+1\rangle

If this is zero, then $j(j+1)=m_{max}(m_{max}+1)$ , so $j=m$ or $j=-m-1$ . However, because $J^{2}-J_{z}^{2}$ is positive-semidefinite, $\hbar ^{2}j(j+1)\geq (\hbar m)^{2}$ , which means that the only possibility is $m_{max}=j$ .

Similarly, consider the first nonzero entry on the left of the ladder, $|j,m_{min}\rangle$ . This state has the property that $J_{-}|j,m_{min}\rangle =0$ . As proven in the ladder operator article,

J_{-}|j,m\rangle =\hbar {\sqrt {j(j+1)-m(m-1)}}|j,m-1\rangle

As above, the only possibility is that $m_{min}=-j$

Since m changes by 1 on each step of the ladder, $(j-(-j))$ is an integer, so j is an integer or half-integer (0 or 0.5 or 1 or 1.5...).

Since S and L have the same commutation relations as J, the same ladder analysis works for them.

The ladder-operator analysis does not explain one aspect of the quantization rules above: the fact that L (unlike J and S) cannot have half-integer quantum numbers. This fact can be proven (at least in the special case of one particle) by writing down every possible eigenfunction of L² and L_z, (they are the spherical harmonics), and seeing explicitly that none of them have half-integer quantum numbers.^[11] An alternative derivation is below.

Visual interpretation

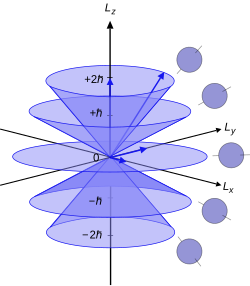
Mining Engineer (Excluding Oil ) Truman from Alma, loves to spend time knotting, largest property developers in singapore developers in singapore and stamp collecting. Recently had a family visit to Urnes Stave Church. Since the angular momenta are quantum operators, they cannot be drawn as vectors like in classical mechanics. Nevertheless, it is common to depict them heuristically in this way. Depicted on the right is a set of states with quantum numbers $\ell =2$ , and $m_{\ell }=-2,-1,0,1,2$ for the five cones from bottom to top. Since $|L|={\sqrt {L^{2}}}=\hbar {\sqrt {6}}$ , the vectors are all shown with length $\hbar {\sqrt {6}}$ . The rings represent the fact that $L_{z}$ is known with certainty, but $L_{x}$ and $L_{y}$ are unknown; therefore every classical vector with the appropriate length and z-component is drawn, forming a cone. The expected value of the angular momentum for a given ensemble of systems in the quantum state characterized by $\ell$ and $m_{\ell }$ could be somewhere on this cone while it cannot be defined for a single system (since the components of $L$ do not commute with each other).

Quantization in macroscopic systems

The quantization rules are technically true even for macroscopic systems, like the angular momentum L of a spinning tire. However they have no observable effect. For example, if $L_{z}/\hbar$ is roughly 100000000, it makes essentially no difference whether the precise value is an integer like 100000000 or 100000001, or a non-integer like 100000000.2—the discrete steps are too small to notice.

Angular momentum as the generator of rotations

DTZ's public sale group in Singapore auctions all forms of residential, workplace and retail properties, outlets, homes, lodges, boarding homes, industrial buildings and development websites. Auctions are at present held as soon as a month.

We will not only get you a property at a rock-backside price but also in an space that you've got longed for. You simply must chill out back after giving us the accountability. We will assure you 100% satisfaction. Since we now have been working in the Singapore actual property market for a very long time, we know the place you may get the best property at the right price. You will also be extremely benefited by choosing us, as we may even let you know about the precise time to invest in the Singapore actual property market.

The Hexacube is offering new ec launch singapore business property for sale Singapore investors want to contemplate. Residents of the realm will likely appreciate that they'll customize the business area that they wish to purchase as properly. This venture represents one of the crucial expansive buildings offered in Singapore up to now. Many investors will possible want to try how they will customise the property that they do determine to buy by means of here. This location has offered folks the prospect that they should understand extra about how this course of can work as well.

Singapore has been beckoning to traders ever since the value of properties in Singapore started sky rocketing just a few years again. Many businesses have their places of work in Singapore and prefer to own their own workplace area within the country once they decide to have a everlasting office. Rentals in Singapore in the corporate sector can make sense for some time until a business has discovered a agency footing. Finding Commercial Property Singapore takes a variety of time and effort but might be very rewarding in the long term.

is changing into a rising pattern among Singaporeans as the standard of living is increasing over time and more Singaporeans have abundance of capital to invest on properties. Investing in the personal properties in Singapore I would like to applaud you for arising with such a book which covers the secrets and techniques and tips of among the profitable Singapore property buyers. I believe many novice investors will profit quite a bit from studying and making use of some of the tips shared by the gurus." – Woo Chee Hoe Special bonus for consumers of Secrets of Singapore Property Gurus Actually, I can't consider one other resource on the market that teaches you all the points above about Singapore property at such a low value. Can you? Condominium For Sale (D09) – Yong An Park For Lease

In 12 months 2013, c ommercial retails, shoebox residences and mass market properties continued to be the celebrities of the property market. Models are snapped up in report time and at document breaking prices. Builders are having fun with overwhelming demand and patrons need more. We feel that these segments of the property market are booming is a repercussion of the property cooling measures no.6 and no. 7. With additional buyer's stamp responsibility imposed on residential properties, buyers change their focus to commercial and industrial properties. I imagine every property purchasers need their property funding to understand in value. The most general and fundamental definition of angular momentum is as the generator of rotations.^[6] More specifically, let $R({\hat {n}},\phi )$ be a rotation operator, which rotates any quantum state about axis ${\hat {n}}$ by angle $\phi$ . As $\phi \rightarrow 0$ , the operator $R({\hat {n}},\phi )$ approaches the identity operator, because a rotation of 0° maps all states to themselves. Then the angular momentum operator $J_{\hat {n}}$ about axis ${\hat {n}}$ is defined as:^[6]

J_{\hat {n}}\equiv i\hbar \lim _{\phi \rightarrow 0}{\frac {R({\hat {n}},\phi )-1}{\phi }}

where 1 is the identity operator. Remark also that R is an additive morphism : $R({\hat {n}},\phi _{1}+\phi _{2})=R({\hat {n}},\phi _{1})R({\hat {n}},\phi _{2})$ ; as a consequence^[6]

R({\hat {n}},\phi )=\exp(-i\phi J_{\hat {n}}/\hbar )

where exp is matrix exponential.

In simpler terms, the total angular momentum operator characterizes how a quantum system is changed when it is rotated. The relationship between angular momentum operators and rotation operators is the same as the relationship between Lie algebras and Lie groups in mathematics, as discussed further below.

The different types of rotation operators. Top: Two particles, with spin states indicated schematically by the arrows. (A) The operator R, related to J, rotates the entire system. (B) The operator R_spatial, related to L, rotates the particle positions without altering their internal spin states. (C) The operator R_internal, related to S, rotates the particles' internal spin states without changing their positions.

Just as J is the generator for rotation operators, L and S are generators for modified partial rotation operators. The operator

R_{\mathrm {spatial} }({\hat {n}},\phi )=\exp(-i\phi L_{\hat {n}}/\hbar ),

rotates the position (in space) of all particles and fields, without rotating the internal (spin) state of any particle. Likewise, the operator

R_{\mathrm {internal} }({\hat {n}},\phi )=\exp(-i\phi S_{\hat {n}}/\hbar ),

rotates the internal (spin) state of all particles, without moving any particles or fields in space. The relation J=L+S comes from:

R({\hat {n}},\phi )=R_{\mathrm {internal} }({\hat {n}},\phi )R_{\mathrm {spatial} }({\hat {n}},\phi )

i.e. if the positions are rotated, and then the internal states are rotated, then altogether the complete system has been rotated.

SU(2), SO(3), and 360° rotations

Mining Engineer (Excluding Oil ) Truman from Alma, loves to spend time knotting, largest property developers in singapore developers in singapore and stamp collecting. Recently had a family visit to Urnes Stave Church. Although one might expect $R({\hat {n}},360^{\circ })=1$ (a rotation of 360° is the identity operator), this is not assumed in quantum mechanics, and it turns out it is often not true: When the total angular momentum quantum number is a half-integer (1/2, 3/2, etc.), $R({\hat {n}},360^{\circ })=-1$ , and when it is an integer, $R({\hat {n}},360^{\circ })=+1$ .^[6] Mathematically, the structure of rotations in the universe is not SO(3), the group of three-dimensional rotations in classical mechanics. Instead, it is SU(2), which is identical to SO(3) for small rotations, but where a 360° rotation is mathematically distinguished from a rotation of 0°. (A rotation of 720° is, however, the same as a rotation of 0°.)^[6]

On the other hand, $R_{\mathrm {spatial} }({\hat {n}},360^{\circ })=+1$ in all circumstances, because a 360° rotation of a spatial configuration is the same as no rotation at all. (This is different from a 360° rotation of the internal (spin) state of the particle, which might or might not be the same as no rotation at all.) In other words, the $R_{\mathrm {spatial} }$ operators carry the structure of SO(3), while $R$ and $R_{\mathrm {internal} }$ carry the structure of SU(2).

From the equation $+1=R_{\mathrm {spatial} }({\hat {z}},360^{\circ })=\exp(-2\pi iL_{z}/\hbar )$ , one picks an eigenstate $L_{z}|\psi \rangle =m\hbar |\psi \rangle$ and draws

e^{-2\pi im}=1

which is to say that the orbital angular momentum quantum numbers can only be integers, not half-integers.

Connection to representation theory

Mining Engineer (Excluding Oil ) Truman from Alma, loves to spend time knotting, largest property developers in singapore developers in singapore and stamp collecting. Recently had a family visit to Urnes Stave Church. Starting with a certain quantum state $|\psi _{0}\rangle$ , consider the set of states $R({\hat {n}},\phi )|\psi _{0}\rangle$ for all possible ${\hat {n}}$ and $\phi$ , i.e. the set of states that come about from rotating the starting state in every possible way. This is a vector space, and therefore the manner in which the rotation operators map one state onto another is a representation of the group of rotation operators.

When rotation operators act on quantum states, it forms a representation of the Lie group SU(2) (for R and R_internal), or SO(3) (for R_spatial).

From the relation between J and rotation operators,

When angular momentum operators act on quantum states, it forms a representation of the Lie algebra SU(2) or SO(3).

(The Lie algebras of SU(2) and SO(3) are identical.)

The ladder operator derivation above is a method for classifying the representations of the Lie algebra SU(2).

Connection to commutation relations

Classical rotations do not commute with each other: For example, rotating 1° about the x-axis then 1° about the y-axis gives a slightly different overall rotation than rotating 1° about the y-axis then 1° about the x-axis. By carefully analyzing this noncommutativity, the commutation relations of the angular momentum operators can be derived.^[6]

(This same calculational procedure is one way to answer the mathematical question "What is the Lie algebra of the Lie groups SO(3) or SU(2)?")

Conservation of angular momentum

The Hamiltonian H represents the energy and dynamics of the system. In a spherically-symmetric situation, the Hamiltonian is invariant under rotations:

RHR^{-1}=H

where R is a rotation operator. As a consequence, $[H,R]=0$ , and then $[H,\mathbf {J} ]=0$ due to the relationship between J and R. By the Ehrenfest theorem, it follows that J is conserved.

To summarize, if H is rotationally-invariant (spherically symmetric), then total angular momentum J is conserved. This is an example of Noether's theorem.

If H is just the Hamiltonian for one particle, the total angular momentum of that one particle is conserved when the particle is in a central potential (i.e., when the potential energy function depends only on $|\mathbf {r} |$ ). Alternatively, H may be the Hamiltonian of all particles and fields in the universe, and then H is always rotationally-invariant, as the fundamental laws of physics of the universe are the same regardless of orientation. This is the basis for saying conservation of angular momentum is a general principle of physics.

For a particle without spin, J=L, so orbital angular momentum is conserved in the same circumstances. When the spin is nonzero, the spin-orbit interaction allows angular momentum to transfer from L to S or back. Therefore, L is not, on its own, conserved.

Angular momentum coupling

Mining Engineer (Excluding Oil ) Truman from Alma, loves to spend time knotting, largest property developers in singapore developers in singapore and stamp collecting. Recently had a family visit to Urnes Stave Church.

Often, two or more sorts of angular momentum interact with each other, so that angular momentum can transfer from one to the other. For example, in spin-orbit coupling, angular momentum can transfer between L and S, but only the total J=L+S is conserved. In another example, in an atom with two electrons, each has its own angular momentum J₁ and J₂, but only the total J=J₁+J₂ is conserved.

In these situations, it is often useful to know the relationship between, on the one hand, states where $(J_{1})_{z},(J_{1})^{2},(J_{2})_{z},(J_{2})^{2}$ all have definite values, and on the other hand, states where $(J_{1})^{2},(J_{2})^{2},J^{2},J_{z}$ all have definite values, as the latter four are usually conserved (constants of motion). The procedure to go back and forth between these bases is to use Clebsch–Gordan coefficients.

One important result in this field is that a relationship between the quantum numbers for $(J_{1})^{2},(J_{2})^{2},J^{2}$ :

j\in \{|j_{1}-j_{2}|,(|j_{1}-j_{2}|+1),\ldots ,(j_{1}+j_{2})\}

.

For an atom or molecule with J = L + S, the term symbol gives the quantum numbers associated with the operators $L^{2},S^{2},J^{2}$ .

Orbital angular momentum in spherical coordinates

Angular momentum operators usually occur when solving a problem with spherical symmetry in spherical coordinates. The angular momentum in space representation is ^[12]

L_{x}=i\hbar \left(\sin \phi {\frac {\partial }{\partial \theta }}+\cot \theta \cos \phi {\frac {\partial }{\partial \phi }}\right),

L_{y}=i\hbar \left(-\cos \phi {\frac {\partial }{\partial \theta }}+\cot \theta \sin \phi {\frac {\partial }{\partial \phi }}\right),

L_{z}=-i\hbar {\frac {\partial }{\partial \phi ,}}

and

L^{2}=-\hbar ^{2}\left({\frac {1}{\sin \theta }}{\frac {\partial }{\partial \theta }}\left(\sin \theta {\frac {\partial }{\partial \theta }}\right)+{\frac {1}{\sin ^{2}\theta }}{\frac {\partial ^{2}}{\partial \phi ^{2}}}\right).

When solving to find eigenstates of this operator, we obtain the following

L^{2}\mid l,m\rangle ={\hbar }^{2}l(l+1)|l,m\rangle

L_{z}\mid l,m\rangle =\hbar m|l,m\rangle

where

\langle \theta ,\phi |l,m\rangle =Y_{l,m}(\theta ,\phi )

are the spherical harmonics.

References

↑ Introductory Quantum Mechanics, Richard L. Liboff, 2nd Edition, ISBN 0-201-54715-5
↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.

My blog: http://www.primaboinca.com/view_profile.php?userid=5889534
↑ H. Goldstein, C. P. Poole and J. Safko, Classical Mechanics, 3rd Edition, Addison-Wesley 2002, pp. 388 ﬀ.
↑ Idem, pp. 408-411.
↑ Idem, p. 404.
↑ ^6.0 ^6.1 ^6.2 ^6.3 ^6.4 ^6.5 ^6.6 Template:Cite web
↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.

My blog: http://www.primaboinca.com/view_profile.php?userid=5889534
↑ Goldstein et al, p. 410
↑ Introduction to quantum mechanics: with applications to chemistry, by Linus Pauling, Edgar Bright Wilson, page 45, google books link
↑ ^10.0 ^10.1 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.

My blog: http://www.primaboinca.com/view_profile.php?userid=5889534
↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.

My blog: http://www.primaboinca.com/view_profile.php?userid=5889534
↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.

My blog: http://www.primaboinca.com/view_profile.php?userid=5889534