Positron emission tomography: Difference between revisions
en>Sfan00 IMG Filling in 3 references using Reflinks |
en>EdJohnston Change some occurrences of 'PET/' to 'PET-' per the result of the move discussion at Talk:PET-CT#Move? |
||
| Line 1: | Line 1: | ||
[[File:InfiniteSquareWellAnimation.gif|thumb|300px|right|Some trajectories of a particle in a box according to [[Newton's laws]] of [[classical mechanics]] (A), and according to the [[Schrödinger equation]] of [[quantum mechanics]] (B-F). In (B-F), the horizontal axis is position, and the vertical axis is the real part (blue) and imaginary part (red) of the [[wavefunction]]. The states (B,C,D) are [[energy eigenstate]]s, but (E,F) are not.]] | |||
In [[quantum mechanics]], the '''particle in a box''' model (also known as the '''infinite potential well''' or the '''infinite square well''') describes a particle free to move in a small space surrounded by impenetrable barriers. The model is mainly used as a hypothetical example to illustrate the differences between [[classical physics|classical]] and quantum systems. In classical systems, for example a ball trapped inside a large box, the particle can move at any speed within the box and it is no more likely to be found at one position than another. However, when the well becomes very narrow (on the scale of a few [[nanometer]]s), quantum effects become important. The particle may only occupy certain positive [[energy levels]]. Likewise, it can never have zero energy, meaning that the particle can never "sit still". Additionally, it is more likely to be found at certain positions than at others, depending on its energy level. The particle may never be detected at certain positions, known as spatial nodes. | |||
The particle in a box model provides one of the very few problems in quantum mechanics which can be solved analytically, without approximations. This means that the observable properties of the particle (such as its energy and position) are related to the mass of the particle and the width of the well by simple mathematical expressions. Due to its simplicity, the model allows insight into quantum effects without the need for complicated mathematics. It is one of the first quantum mechanics problems taught in undergraduate physics courses, and it is commonly used as an approximation for more complicated quantum systems. See also: the [[history of quantum mechanics]]. | |||
== One-dimensional solution == | |||
[[File:Infinite potential well-en.svg|thumb|right|The barriers outside a one-dimensional box have infinitely large potential, while the interior of the box has a constant, zero potential.]] | |||
The simplest form of the particle in a box model considers a one-dimensional system. Here, the particle may only move backwards and forwards along a straight line with impenetrable barriers at either end.<ref name="Davies4">Davies, p.4</ref> | |||
The walls of a one-dimensional box may be visualised as regions of space with an infinitely large [[potential energy]]. Conversely, the interior of the box has a constant, zero potential energy.<ref>Actually, any constant, finite potential <math>V_0</math> can be specified within the box. This merely shifts the energies of the states by <math>V_0</math>.</ref> This means that no forces act upon the particle inside the box and it can move freely in that region. However, infinitely large [[force]]s repel the particle if it touches the walls of the box, preventing it from escaping. The potential energy in this model is given as | |||
:<math>V(x) = | |||
\begin{cases} | |||
0, & 0 < x < L,\\ | |||
\infty, & \text{otherwise,} | |||
\end{cases}, | |||
</math> | |||
where <math>L</math> is the length of the box and <math>x</math> is the position of the particle within the box. | |||
=== Wavefunctions === | |||
In quantum mechanics, the [[wavefunction]] gives the most fundamental description of the behavior of a particle; the measurable properties of the particle (such as its position, momentum and energy) may all be derived from the wavefunction.<ref name="Davies1">Davies, p. 1</ref> | |||
The wavefunction <math>\psi(x,t)</math> can be found by solving the [[Schrödinger equation]] for the system | |||
:<math>\mathrm{i}\hbar\frac{\partial}{\partial t}\psi(x,t) = -\frac{\hbar^2}{2m}\frac{\partial^2}{\partial x^2}\psi(x,t) +V(x)\psi(x,t),</math> | |||
where <math>\hbar</math> is the [[reduced Planck constant]], <math>m</math> is the [[mass]] of the particle, <math>\mathrm{i}</math> is the [[imaginary unit]] and <math>t</math> is time. | |||
Inside the box, no forces act upon the particle, which means that the part of the wavefunction inside the box oscillates through space and time with the same form as a [[free particle]]:<ref name="Davies4" /><ref name = "Bransden157">Bransden and Joachain, p. 157</ref> | |||
:<math>\psi(x,t) = [A \sin(kx) + B \cos(kx)]\mathrm{e}^{-\mathrm{i}\omega t},</math> | |||
where <math>A</math> and <math>B</math> are arbitrary [[complex number]]s. The frequency of the oscillations through space and time are given by the [[wavenumber]] <math>k</math> and the [[angular frequency]] <math>\omega</math> respectively. These are both related to the total energy of the particle by the expression | |||
:<math>E = \hbar\omega = \frac{\hbar^2 k^2}{2m},</math> | |||
which is known as the [[dispersion relation]] for a free particle.<ref name = "Davies4" /> | |||
[[File:particle in a box wavefunctions 2.svg|thumb|right|upright|Initial wavefunctions for the first four states in a one-dimensional particle in a box]] | |||
The size (or [[amplitude]]) of the wavefunction at a given position is related to the probability of finding a particle there by <math>P(x,t) = |\psi(x,t)|^2</math>. The wavefunction must therefore vanish everywhere beyond the edges of the box.<ref name="Davies4" /><ref name="Bransden157" /> Also, the amplitude of the wavefunction may not "jump" abruptly from one point to the next.<ref name="Davies4" /> These two conditions are only satisfied by wavefunctions with the form | |||
:<math>\psi_n(x,t) = | |||
\begin{cases} | |||
A \sin(k_n x)\mathrm{e}^{-\mathrm{i}\omega_n t}, & 0 < x < L,\\ | |||
0, & \text{otherwise,} | |||
\end{cases} | |||
</math> | |||
where <math>n</math> is a positive integer. The wavenumber is restricted to certain, specific values given by<ref name = "Davies5">Davies p. 5</ref> | |||
:<math>k_n = \frac{n \pi}{L}, \quad \mathrm{where} \quad n = \{1,2,3,4,\ldots\},</math> | |||
where <math>L</math> is the size of the box.{{#tag:ref|The simplest solutions, <math>k_n=0</math> or <math>A=0</math> both yield the trivial wavefunction <math>\psi(x)=0</math>, which describes a particle that does not exist anywhere in the system.<ref name="Bransden158">Bransden and Joachain, p.158</ref>}} Negative values of <math>n</math> are neglected, since they give wavefunctions identical to the positive <math>n</math> solutions except for a physically unimportant sign change.<ref name="Bransden158" /> | |||
Finally, the unknown constant <math>A</math> may be found by [[Normalizable wavefunction|normalizing the wavefunction]] so that the total probability density of finding the particle in the system is 1. It follows that | |||
:<math>\left| A \right| = \sqrt{\frac{2 }{L}}.</math> | |||
Thus, ''A'' may be any complex number with [[absolute value]] √(2/L); these different values of ''A'' yield the same physical state, so ''A'' = √(2/L) can be selected to simplify. | |||
The above solution is for the specific case of a box situated between <math>0</math> and <math>L</math>. It's expected that the ''eigenvalues'', i.e., the energy <math>E_n</math> of the box should be the same regardless of its position in space, but <math>\psi_n(x,t)</math> changes. This is represented by a more general case of <math>\psi_n(x,t)</math>: | |||
:<math>\psi_n(x,t) = | |||
\begin{cases} | |||
\sqrt{\frac{2 }{L}} \sin(k_n x - \frac{n \pi x_0}{L})\mathrm{e}^{-\mathrm{i}\omega_n t}, & x_0 < x < x_0 + L,\\ | |||
0, & \text{otherwise,} | |||
\end{cases} | |||
</math> | |||
Where <math>x_0</math> is the initial position. Notice that <math>\frac{n \pi x_0}{L}</math> represents a phase shift in the wave function, and <math>\psi_n(x,t)</math> simplifies to the above case when <math>x_0 = 0</math>. Also, the phase shift has no effect when solving the Schrödinger equation, thus not affecting the ''eigenvalue''. | |||
The momentum wavefunction is proportional to the [[Fourier transform]] of the position wavefunction. With <math>k=p/\hbar</math> and <math>\omega_n=\frac{\pi h n^2}{4 L^2 m}</math>, | |||
:<math>\phi_n(p,t)=\frac{1}{\sqrt{2\pi\hbar}}\int_{-\infty}^\infty \psi_n(x,t)e^{-ikx}\,dx = | |||
\sqrt{\frac{\pi L}{\hbar}}\,\,\frac{n\left(1-(-1)^ne^{-ikL}\right) e^{-i \omega_n t}}{\pi ^2 n^2-k^2 L^2}</math> | |||
=== Position and momentum === | |||
In classical physics, the particle can be detected anywhere in the box with equal probability. In quantum mechanics, however, the probability density for finding a particle at a given position is derived from the wavefunction as <math>P(x) = |\psi(x)|^2.</math> For the particle in a box, the probability density for finding the particle at a given position depends upon its state, and is given by | |||
:<math>P_n(x) = | |||
\begin{cases} | |||
\frac{2 }{L}\sin^2\left(\frac{n\pi x}{L}\right); & 0 < x < L \\ | |||
0; & \text{otherwise}. | |||
\end{cases} | |||
</math> | |||
Thus, for any value of ''n'' greater than one, there are regions within the box for which <math>P(x)=0</math>, indicating that ''spatial nodes'' exist at which the particle cannot be found. | |||
In quantum mechanics, the average, or [[expectation value]] of the position of a particle is given by | |||
:<math>\langle x \rangle = \int_{-\infty}^{\infty} x P_n(x)\,\mathrm{d}x.</math> | |||
For the steady state particle in a box, it can be shown that the average position is always <math>\langle x \rangle = L/2</math>, regardless of the state of the particle. For a superposition of states, the expectation value of the position will change based on the cross term which is proportional to <math>cos(\omega t)</math>. | |||
The variance in the position is a measure of the uncertainty in position of the particle: | |||
:<math>\mathrm{Var}(x)=\int_{-\infty}^\infty (x-\langle x\rangle)^2 P_n(x)\,dx = \frac{L^2}{12}\left(1-\frac{6}{n^2\pi^2}\right)</math> | |||
The probability density for finding a particle with a given momentum is derived from the wavefunction as <math>P(x) = |\phi(x)|^2.</math>. As with position, the probability density for finding the particle at a given momentum depends upon its state, and is given by | |||
:<math>P_n(p)=\frac{2 \pi L}{\hbar}\, \frac{n^2 \left(1-(-1)^n \cos (k L)\right)}{\left(k^2 L^2-\pi ^2 n^2\right)^2}</math> | |||
where, again, <math>k=p/\hbar</math>. The expectation value for the momentum is then calculated to be zero, and the variance in the momentum is calculated to be: | |||
:<math>\mathrm{Var}(p)=\left(\frac{\hbar n\pi}{L}\right)^2</math> | |||
The uncertainties in position and momentum (<math>\Delta x</math> and <math>\Delta p</math>) are defined as being equal to the square root of their respective variances, so that: | |||
:<math>\Delta x \Delta p = \frac{\hbar}{2} \sqrt{\frac{n^2\pi^2}{3}-2}</math> | |||
This product increases with increasing ''n'', having a minimum value for ''n=1''. The value of this product for ''n=1'' is about equal to 0.568 <math>\hbar</math> which obeys the [[Heisenberg uncertainty principle]], which states that the product will be greater than or equal to <math>\hbar/2</math> | |||
=== Energy levels === | |||
[[File:Confined particle dispersion - positive.svg|thumb|upright|The energy of a particle in a box (black circles) and a free particle (grey line) both depend upon wavenumber in the same way. However, the particle in a box may only have certain, discrete energy levels.]] | |||
The energies which correspond with each of the permitted wavenumbers may be written as<ref name="Davies5" /> | |||
:<math>E_n = \frac{n^2\hbar^2 \pi ^2}{2mL^2} = \frac{n^2 h^2}{8mL^2}</math>. | |||
The energy levels increase with <math>n^2</math>, meaning that high energy levels are separated from each other by a greater amount than low energy levels are. The lowest possible energy for the particle (its ''[[zero-point energy]]'') is found in state 1, which is given by<ref name="Bransden159">Bransden and Joachain, p. 159</ref> | |||
:<math>E_1 = \frac{\hbar^2\pi^2}{2mL^2}.</math> | |||
The particle, therefore, always has a positive energy. This contrasts with classical systems, where the particle can have zero energy by resting motionless at the bottom of the box. This can be explained in terms of the [[uncertainty principle]], which states that the product of the uncertainties in the position and momentum of a particle is limited by | |||
:<math>\Delta x\Delta p \geq \frac{\hbar}{2}</math> | |||
It can be shown that the uncertainty in the position of the particle is proportional to the width of the box.<ref name="Davies15">Davies, p. 15</ref> Thus, the uncertainty in momentum is roughly inversely proportional to the width of the box.<ref name="Bransden159" /> The kinetic energy of a particle is given by <math>E=p^2/(2m)</math>, and hence the minimum kinetic energy of the particle in a box is inversely proportional to the mass and the square of the well width, in qualitative agreement with the calculation above.<ref name="Bransden159" /> | |||
==Higher-dimensional boxes== | |||
[[Image:Particle2D.svg|thumb|320px|right|The wavefunction of a 2D well with n<sub>x</sub>=4 and n<sub>y</sub>=4]] | |||
If a particle is trapped in a two-dimensional box, it may freely move in the <math>x</math> and <math>y</math>-directions, between barriers separated by lengths <math>L_x</math> and <math>L_y</math> respectively. Using a similar approach to that of the one-dimensional box, it can be shown that the wavefunctions and energies are given respectively by | |||
:<math>\psi_{n_x,n_y} = \sqrt{\frac{4}{L_x L_y}} \sin \left( k_{n_x} x \right) \sin \left( k_{n_y} y\right)</math>, | |||
:<math>E_{n_x,n_y} = \frac{\hbar^2 k_{n_x,n_y}^2}{2m}</math>, | |||
where the two-dimensional [[wavevector]] is given by | |||
:<math>\mathbf{k_{n_x,n_y}} = k_{n_x}\mathbf{\hat{x}} + k_{n_y}\mathbf{\hat{y}} = \frac{n_x \pi }{L_x} \mathbf{\hat{x}} + \frac{n_y \pi }{L_y} \mathbf{\hat{y}}</math>. | |||
For a three dimensional box, the solutions are | |||
:<math>\psi_{n_x,n_y,n_z} = \sqrt{\frac{8}{L_x L_y L_z}} \sin \left( k_{n_x} x \right) \sin \left( k_{n_y} y \right) \sin \left( k_{n_z} z \right)</math>, | |||
:<math>E_{n_x,n_y,n_z} = \frac{\hbar^2 k_{n_x,n_y,n_z}^2}{2m}</math>, | |||
where the three-dimensional wavevector is given by | |||
:<math>\mathbf{k_{n_x,n_y,n_z}} = k_{n_x}\mathbf{\hat{x}} + k_{n_y}\mathbf{\hat{y}} + k_{n_z}\mathbf{\hat{z}} = \frac{n_x \pi }{L_x} \mathbf{\hat{x}} + \frac{n_y \pi }{L_y} \mathbf{\hat{y}} + \frac{n_z \pi }{L_z} \mathbf{\hat{z}}</math>. | |||
In general for an n-dimensional box, the solutions are | |||
:<math> \psi = \sqrt{\frac{2^n}{\prod_{i} L_i}} \prod_{i}\sin(k_i i)</math> | |||
An interesting feature of the above solutions is that when two or more of the lengths are the same (e.g. <math>L_x = L_y</math>), there are multiple wavefunctions corresponding to the same total energy. For example the wavefunction with <math>n_x = 2, n_y = 1</math> has the same energy as the wavefunction with <math>n_x = 1, n_y = 2</math>. This situation is called ''[[Degenerate energy level|degeneracy]]'' and for the case where exactly two degenerate wavefunctions have the same energy that energy level is said to be ''doubly degenerate''. Degeneracy results from symmetry in the system. For the above case two of the lengths are equal so the system is symmetric with respect to a 90° rotation. | |||
== Applications == | |||
{{Expand section|date=January 2010}} | |||
Because of its mathematical simplicity, the particle in a box model is used to find approximate solutions for more complex physical systems in which a particle is trapped in a narrow region of low [[electric potential]] between two high potential barriers. These [[quantum well]] systems are particularly important in [[optoelectronics]], and are used in devices such as the [[quantum well laser]], the [[quantum well infrared photodetector]] and the [[quantum-confined Stark effect]] modulator. | |||
== Relativistic Effects == | |||
{{Expand section|date=August 2013}} | |||
The probability density does not go to zero at the nodes if relativistic effects are taken into account.<ref>{{cite journal|doi=10.1088/0143-0807/17/1/004 |title=Relativistic particle in a box |year=1996 |last1=Alberto |first1=P |last2=Fiolhais |first2=C |last3=Gil |first3=V M S |url=https://estudogeral.sib.uc.pt/bitstream/10316/12349/1/Relativistic%20particle%20in%20a%20box.pdf |format=PDF |journal=European Journal of Physics |volume=17 |pages=19–24 |bibcode=1996EJPh...17...19A}}</ref> | |||
==See also== | |||
* [[Finite potential well]] | |||
* [[Delta function potential]] | |||
* [[Gas in a box]] | |||
* [[Particle in a ring]] | |||
* [[Particle in a spherically symmetric potential]] | |||
* [[Quantum harmonic oscillator]] | |||
* [[Delta potential well (QM)]] | |||
* [[Semicircle potential well]] | |||
* [[Configuration integral]] (statistical mechanics) | |||
== References == | |||
{{reflist|2}} | |||
== Bibliography == | |||
*{{cite book | last1=Bransden | first1=B. H. | last2 = Joachain | first2 = C. J. | title = Quantum mechanics | edition = 2nd | publisher = Pearson Education|location=Essex| year = 2000|isbn=0-582-35691-1}} | |||
*{{cite book | last=Davies|first= John H.|title=The Physics of Low-Dimensional Semiconductors: An Introduction | publisher=Cambridge University Press|year=2006|isbn=0-521-48491-X|edition=6th reprint}} | |||
*{{cite book | last=Griffiths|first= David J.|title=Introduction to Quantum Mechanics |edition=2nd | publisher=Prentice Hall |year=2004 |isbn=0-13-111892-7}} | |||
==External links== | |||
* [http://scienceworld.wolfram.com/physics/InfiniteSquarePotentialWell.html Scienceworld] (Infinite Potential Well) | |||
* [http://www.falstad.com/qm1d/ 1-D quantum mechanics java applet] simulates particle in a box, as well as other 1-dimensional cases. | |||
* [http://www.falstad.com/qm2dbox/ 2-D particle in a box applet] | |||
{{DEFAULTSORT:Particle In A Box}} | |||
[[Category:Quantum models]] | |||
Revision as of 19:09, 15 January 2014

In quantum mechanics, the particle in a box model (also known as the infinite potential well or the infinite square well) describes a particle free to move in a small space surrounded by impenetrable barriers. The model is mainly used as a hypothetical example to illustrate the differences between classical and quantum systems. In classical systems, for example a ball trapped inside a large box, the particle can move at any speed within the box and it is no more likely to be found at one position than another. However, when the well becomes very narrow (on the scale of a few nanometers), quantum effects become important. The particle may only occupy certain positive energy levels. Likewise, it can never have zero energy, meaning that the particle can never "sit still". Additionally, it is more likely to be found at certain positions than at others, depending on its energy level. The particle may never be detected at certain positions, known as spatial nodes.
The particle in a box model provides one of the very few problems in quantum mechanics which can be solved analytically, without approximations. This means that the observable properties of the particle (such as its energy and position) are related to the mass of the particle and the width of the well by simple mathematical expressions. Due to its simplicity, the model allows insight into quantum effects without the need for complicated mathematics. It is one of the first quantum mechanics problems taught in undergraduate physics courses, and it is commonly used as an approximation for more complicated quantum systems. See also: the history of quantum mechanics.
One-dimensional solution
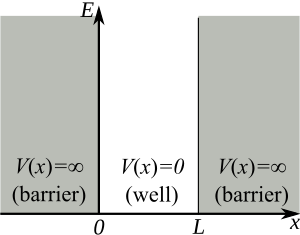
The simplest form of the particle in a box model considers a one-dimensional system. Here, the particle may only move backwards and forwards along a straight line with impenetrable barriers at either end.[1] The walls of a one-dimensional box may be visualised as regions of space with an infinitely large potential energy. Conversely, the interior of the box has a constant, zero potential energy.[2] This means that no forces act upon the particle inside the box and it can move freely in that region. However, infinitely large forces repel the particle if it touches the walls of the box, preventing it from escaping. The potential energy in this model is given as
where is the length of the box and is the position of the particle within the box.
Wavefunctions
In quantum mechanics, the wavefunction gives the most fundamental description of the behavior of a particle; the measurable properties of the particle (such as its position, momentum and energy) may all be derived from the wavefunction.[3] The wavefunction can be found by solving the Schrödinger equation for the system
where is the reduced Planck constant, is the mass of the particle, is the imaginary unit and is time.
Inside the box, no forces act upon the particle, which means that the part of the wavefunction inside the box oscillates through space and time with the same form as a free particle:[1][4]
where and are arbitrary complex numbers. The frequency of the oscillations through space and time are given by the wavenumber and the angular frequency respectively. These are both related to the total energy of the particle by the expression
which is known as the dispersion relation for a free particle.[1]

The size (or amplitude) of the wavefunction at a given position is related to the probability of finding a particle there by . The wavefunction must therefore vanish everywhere beyond the edges of the box.[1][4] Also, the amplitude of the wavefunction may not "jump" abruptly from one point to the next.[1] These two conditions are only satisfied by wavefunctions with the form
where is a positive integer. The wavenumber is restricted to certain, specific values given by[5]
where is the size of the box.[7] Negative values of are neglected, since they give wavefunctions identical to the positive solutions except for a physically unimportant sign change.[6]
Finally, the unknown constant may be found by normalizing the wavefunction so that the total probability density of finding the particle in the system is 1. It follows that
Thus, A may be any complex number with absolute value √(2/L); these different values of A yield the same physical state, so A = √(2/L) can be selected to simplify.
The above solution is for the specific case of a box situated between and . It's expected that the eigenvalues, i.e., the energy of the box should be the same regardless of its position in space, but changes. This is represented by a more general case of :
Where is the initial position. Notice that represents a phase shift in the wave function, and simplifies to the above case when . Also, the phase shift has no effect when solving the Schrödinger equation, thus not affecting the eigenvalue.
The momentum wavefunction is proportional to the Fourier transform of the position wavefunction. With and ,
Position and momentum
In classical physics, the particle can be detected anywhere in the box with equal probability. In quantum mechanics, however, the probability density for finding a particle at a given position is derived from the wavefunction as For the particle in a box, the probability density for finding the particle at a given position depends upon its state, and is given by
Thus, for any value of n greater than one, there are regions within the box for which , indicating that spatial nodes exist at which the particle cannot be found.
In quantum mechanics, the average, or expectation value of the position of a particle is given by
For the steady state particle in a box, it can be shown that the average position is always , regardless of the state of the particle. For a superposition of states, the expectation value of the position will change based on the cross term which is proportional to .
The variance in the position is a measure of the uncertainty in position of the particle:
The probability density for finding a particle with a given momentum is derived from the wavefunction as . As with position, the probability density for finding the particle at a given momentum depends upon its state, and is given by
where, again, . The expectation value for the momentum is then calculated to be zero, and the variance in the momentum is calculated to be:
The uncertainties in position and momentum ( and ) are defined as being equal to the square root of their respective variances, so that:
This product increases with increasing n, having a minimum value for n=1. The value of this product for n=1 is about equal to 0.568 which obeys the Heisenberg uncertainty principle, which states that the product will be greater than or equal to
Energy levels

The energies which correspond with each of the permitted wavenumbers may be written as[5]
The energy levels increase with , meaning that high energy levels are separated from each other by a greater amount than low energy levels are. The lowest possible energy for the particle (its zero-point energy) is found in state 1, which is given by[8]
The particle, therefore, always has a positive energy. This contrasts with classical systems, where the particle can have zero energy by resting motionless at the bottom of the box. This can be explained in terms of the uncertainty principle, which states that the product of the uncertainties in the position and momentum of a particle is limited by
It can be shown that the uncertainty in the position of the particle is proportional to the width of the box.[9] Thus, the uncertainty in momentum is roughly inversely proportional to the width of the box.[8] The kinetic energy of a particle is given by , and hence the minimum kinetic energy of the particle in a box is inversely proportional to the mass and the square of the well width, in qualitative agreement with the calculation above.[8]
Higher-dimensional boxes

If a particle is trapped in a two-dimensional box, it may freely move in the and -directions, between barriers separated by lengths and respectively. Using a similar approach to that of the one-dimensional box, it can be shown that the wavefunctions and energies are given respectively by
where the two-dimensional wavevector is given by
For a three dimensional box, the solutions are
where the three-dimensional wavevector is given by
In general for an n-dimensional box, the solutions are
An interesting feature of the above solutions is that when two or more of the lengths are the same (e.g. ), there are multiple wavefunctions corresponding to the same total energy. For example the wavefunction with has the same energy as the wavefunction with . This situation is called degeneracy and for the case where exactly two degenerate wavefunctions have the same energy that energy level is said to be doubly degenerate. Degeneracy results from symmetry in the system. For the above case two of the lengths are equal so the system is symmetric with respect to a 90° rotation.
Applications
Template:Expand section Because of its mathematical simplicity, the particle in a box model is used to find approximate solutions for more complex physical systems in which a particle is trapped in a narrow region of low electric potential between two high potential barriers. These quantum well systems are particularly important in optoelectronics, and are used in devices such as the quantum well laser, the quantum well infrared photodetector and the quantum-confined Stark effect modulator.
Relativistic Effects
Template:Expand section The probability density does not go to zero at the nodes if relativistic effects are taken into account.[10]
See also
- Finite potential well
- Delta function potential
- Gas in a box
- Particle in a ring
- Particle in a spherically symmetric potential
- Quantum harmonic oscillator
- Delta potential well (QM)
- Semicircle potential well
- Configuration integral (statistical mechanics)
References
43 year old Petroleum Engineer Harry from Deep River, usually spends time with hobbies and interests like renting movies, property developers in singapore new condominium and vehicle racing. Constantly enjoys going to destinations like Camino Real de Tierra Adentro.
Bibliography
- 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534
External links
- Scienceworld (Infinite Potential Well)
- 1-D quantum mechanics java applet simulates particle in a box, as well as other 1-dimensional cases.
- 2-D particle in a box applet
- ↑ 1.0 1.1 1.2 1.3 1.4 Davies, p.4
- ↑ Actually, any constant, finite potential can be specified within the box. This merely shifts the energies of the states by .
- ↑ Davies, p. 1
- ↑ 4.0 4.1 Bransden and Joachain, p. 157
- ↑ 5.0 5.1 Davies p. 5
- ↑ 6.0 6.1 Bransden and Joachain, p.158
- ↑ The simplest solutions, or both yield the trivial wavefunction , which describes a particle that does not exist anywhere in the system.[6]
- ↑ 8.0 8.1 8.2 Bransden and Joachain, p. 159
- ↑ Davies, p. 15
- ↑ One of the biggest reasons investing in a Singapore new launch is an effective things is as a result of it is doable to be lent massive quantities of money at very low interest rates that you should utilize to purchase it. Then, if property values continue to go up, then you'll get a really high return on funding (ROI). Simply make sure you purchase one of the higher properties, reminiscent of the ones at Fernvale the Riverbank or any Singapore landed property Get Earnings by means of Renting
In its statement, the singapore property listing - website link, government claimed that the majority citizens buying their first residence won't be hurt by the new measures. Some concessions can even be prolonged to chose teams of consumers, similar to married couples with a minimum of one Singaporean partner who are purchasing their second property so long as they intend to promote their first residential property. Lower the LTV limit on housing loans granted by monetary establishments regulated by MAS from 70% to 60% for property purchasers who are individuals with a number of outstanding housing loans on the time of the brand new housing purchase. Singapore Property Measures - 30 August 2010 The most popular seek for the number of bedrooms in Singapore is 4, followed by 2 and three. Lush Acres EC @ Sengkang
Discover out more about real estate funding in the area, together with info on international funding incentives and property possession. Many Singaporeans have been investing in property across the causeway in recent years, attracted by comparatively low prices. However, those who need to exit their investments quickly are likely to face significant challenges when trying to sell their property – and could finally be stuck with a property they can't sell. Career improvement programmes, in-house valuation, auctions and administrative help, venture advertising and marketing, skilled talks and traisning are continuously planned for the sales associates to help them obtain better outcomes for his or her shoppers while at Knight Frank Singapore. No change Present Rules
Extending the tax exemption would help. The exemption, which may be as a lot as $2 million per family, covers individuals who negotiate a principal reduction on their existing mortgage, sell their house short (i.e., for lower than the excellent loans), or take part in a foreclosure course of. An extension of theexemption would seem like a common-sense means to assist stabilize the housing market, but the political turmoil around the fiscal-cliff negotiations means widespread sense could not win out. Home Minority Chief Nancy Pelosi (D-Calif.) believes that the mortgage relief provision will be on the table during the grand-cut price talks, in response to communications director Nadeam Elshami. Buying or promoting of blue mild bulbs is unlawful.
A vendor's stamp duty has been launched on industrial property for the primary time, at rates ranging from 5 per cent to 15 per cent. The Authorities might be trying to reassure the market that they aren't in opposition to foreigners and PRs investing in Singapore's property market. They imposed these measures because of extenuating components available in the market." The sale of new dual-key EC models will even be restricted to multi-generational households only. The models have two separate entrances, permitting grandparents, for example, to dwell separately. The vendor's stamp obligation takes effect right this moment and applies to industrial property and plots which might be offered inside three years of the date of buy. JLL named Best Performing Property Brand for second year running
The data offered is for normal info purposes only and isn't supposed to be personalised investment or monetary advice. Motley Fool Singapore contributor Stanley Lim would not personal shares in any corporations talked about. Singapore private home costs increased by 1.eight% within the fourth quarter of 2012, up from 0.6% within the earlier quarter. Resale prices of government-built HDB residences which are usually bought by Singaporeans, elevated by 2.5%, quarter on quarter, the quickest acquire in five quarters. And industrial property, prices are actually double the levels of three years ago. No withholding tax in the event you sell your property. All your local information regarding vital HDB policies, condominium launches, land growth, commercial property and more
There are various methods to go about discovering the precise property. Some local newspapers (together with the Straits Instances ) have categorised property sections and many local property brokers have websites. Now there are some specifics to consider when buying a 'new launch' rental. Intended use of the unit Every sale begins with 10 p.c low cost for finish of season sale; changes to 20 % discount storewide; follows by additional reduction of fiftyand ends with last discount of 70 % or extra. Typically there is even a warehouse sale or transferring out sale with huge mark-down of costs for stock clearance. Deborah Regulation from Expat Realtor shares her property market update, plus prime rental residences and houses at the moment available to lease Esparina EC @ Sengkang









![\psi (x,t)=[A\sin(kx)+B\cos(kx)]{\mathrm {e}}^{{-{\mathrm {i}}\omega t}},](https://wikimedia.org/api/rest_v1/media/math/render/svg/e3401d2e0d3b0b8dce275cb54bc8f5ae8b523331)























































