Bark scale: Difference between revisions
en>Hyacinth {{Acoustics}} |
en>Mark Arsten Adding {{pp-semi-sock}} (TW) |
||
| Line 1: | Line 1: | ||
'''Linear elasticity''' is the mathematical study of how solid objects deform and become internally stressed due to prescribed loading conditions. Linear elasticity models materials as [[Continuum mechanics|continua]]. Linear elasticity is a simplification of the more general [[Finite strain theory|nonlinear theory of elasticity]] and is a branch of [[continuum mechanics]]. The fundamental "linearizing" assumptions of linear elasticity are: [[Infinitesimal strain theory|infinitesimal strains]] or "small" [[Deformation (mechanics)|deformations]] (or strains) and linear relationships between the components of [[Stress (physics)|stress]] and strain. In addition linear elasticity is valid only for stress states that do not produce [[Yield (engineering)|yielding]]. These assumptions are reasonable for many engineering materials and engineering design scenarios. Linear elasticity is therefore used extensively in [[structural analysis]] and engineering design, often with the aid of [[finite element analysis]]. | |||
==Mathematical formulation== | |||
Equations governing a linear elastic [[boundary value problem]] are based on three [[tensor]] [[partial differential equation]]s for the [[conservation of momentum|balance of linear momentum]] and six [[infinitesimal strain]]-[[displacement field (mechanics)|displacement]] relations. The system of differential equations is completed by a set of [[linear equation|linear]] algebraic [[constitutive equations|constitutive relations]]. | |||
=== Direct tensor form === | |||
In direct [[tensor]] form that is independent of the choice of coordinate system, these governing equations are:<ref name=Slau>Slaughter, W. S., (2002), ''The linearized theory of elasticity'', Birkhauser.</ref> | |||
* [[Momentum#Linear_momentum_for_a_system|Equation of motion]], which is an expression of [[Newton's laws of motion#Newton's second law|Newton's second law]]: | |||
:<math>\boldsymbol{\nabla}\cdot\boldsymbol{\sigma} + \mathbf{F} = \rho\ddot{\mathbf{u}} </math> | |||
* [[Deformation (mechanics)#Infinitesimal strain|Strain-displacement]] equations: | |||
:<math>\boldsymbol{\varepsilon} =\tfrac{1}{2} \left[\boldsymbol{\nabla}\mathbf{u}+(\boldsymbol{\nabla}\mathbf{u})^T\right]\,\!</math> | |||
* [[Constitutive equations]]. For elastic materials, [[Hooke's law]] represents the material behavior and relates the unknown stresses and strains. The general equation for Hooke's law is | |||
:<math> \boldsymbol{\sigma} = \mathsf{C}:\boldsymbol{\varepsilon},</math> | |||
where <math>\boldsymbol{\sigma}</math> is the [[stress (mechanics)|Cauchy stress]] [[tensor]], <math>\boldsymbol{\varepsilon}</math> is the [[infinitesimal strain]] tensor, <math>\mathbf{u}</math> is the [[Displacement (vector)|displacement vector]], <math>\mathsf{C}</math> is the fourth-order [[stiffness tensor]], <math>\mathbf{F}</math> is the body force per unit volume, <math>\rho</math> is the mass density, <math>\boldsymbol{\nabla}</math> represents the [[nabla operator]] and <math>(\bullet)^T</math> represents a [[transpose]], <math>\ddot{(\bullet)}</math> represents the second derivative with respect to time, and <math>\mathbf{A}:\mathbf{B} = A_{ij}B_{ij}</math> is the inner product of two second-order tensors (summation over repeated indices is implied). | |||
=== Cartesian coordinate form === | |||
{{Einstein_summation_convention}} | |||
Expressed in terms of components with respect to a rectangular [[Cartesian coordinate]] system, the governing equations of linear elasticity are:<ref name=Slau/> | |||
* [[Cauchy momentum equation|Equation of motion]]: | |||
:<math>\sigma_{ji,j}+ F_i = \rho \partial_{tt} u_i\,\!</math> | |||
:{| class="collapsible collapsed" width="30%" style="text-align:left" | |||
!Engineering notation | |||
|- | |||
|<math>\frac{\partial \sigma_x}{\partial x} + \frac{\partial \tau_{yx}}{\partial y} + \frac{\partial \tau_{zx}}{\partial z} + F_x = \rho \frac{\partial^2 u_x}{\partial t^2}\,\!</math> | |||
<math>\frac{\partial \tau_{xy}}{\partial x} + \frac{\partial \sigma_y}{\partial y} + \frac{\partial \tau_{zy}}{\partial z} + F_y = \rho \frac{\partial^2 u_y}{\partial t^2}\,\!</math> | |||
<math>\frac{\partial \tau_{xz}}{\partial x} + \frac{\partial \tau_{yz}}{\partial y} + \frac{\partial \sigma_z}{\partial z} + F_z = \rho \frac{\partial^2 u_z}{\partial t^2}\,\!</math> | |||
|} | |||
:where the <math>{(\bullet)}_{,j}</math> subscript is a shorthand for <math>\partial{(\bullet)}/\partial x_j</math> and <math>\partial_{tt}</math> indicates <math>\partial^2/\partial t^2</math>, <math> \sigma_{ij}=\sigma_{ji}\,\!</math> is the Cauchy [[Stress (physics)|stress]] tensor, <math> F_i\,\!</math> are the body forces, <math> \rho\,\!</math> is the mass density, and <math> u_i\,\!</math> is the displacement. | |||
:These are 3 [[System of linear equations#Independence|independent]] equations with 6 independent unknowns (stresses). | |||
* [[Deformation (mechanics)#Infinitesimal strain|Strain-displacement]] equations: | |||
:<math>\varepsilon_{ij} =\frac{1}{2} (u_{j,i}+u_{i,j})\,\!</math> | |||
:{| class="collapsible collapsed" width="30%" style="text-align:left" | |||
!Engineering notation | |||
|- | |||
|<math>\epsilon_x=\frac{\partial u_x}{\partial x}\,\!</math> | |||
|<math>\gamma_{xy}=\frac{\partial u_x}{\partial y}+\frac{\partial u_y}{\partial x}\,\!</math> | |||
|- | |||
|<math>\epsilon_y=\frac{\partial u_y}{\partial y}\,\!</math> | |||
|<math>\gamma_{yz}=\frac{\partial u_y}{\partial z}+\frac{\partial u_z}{\partial y}\,\!</math> | |||
|- | |||
|<math>\epsilon_z=\frac{\partial u_z}{\partial z}\,\!</math> | |||
|<math>\gamma_{zx}=\frac{\partial u_z}{\partial x}+\frac{\partial u_x}{\partial z}\,\!</math> | |||
|} | |||
:where <math> \varepsilon_{ij}=\varepsilon_{ji}\,\!</math> is the strain. These are 6 independent equations relating strains and displacements with 9 independent unknowns (strains and displacements). | |||
* [[Constitutive equations]]. The equation for Hooke's law is: | |||
:<math> | |||
\sigma_{ij} = C_{ijkl} \, \varepsilon_{kl} | |||
\,\!</math> | |||
:where <math>C_{ijkl}</math> is the stiffness tensor. These are 6 independent equations relating stresses and strains. The coefficients of the stiffness tensor can always be specified so that <math> C_{ijkl}=C_{klij}=C_{jikl}=C_{ijlk}</math>. | |||
An elastostatic boundary value problem for an isotropic-homogeneous media is a system of 15 independent equations and equal number of unknowns (3 equilibrium equations, 6 strain-displacement equations, and 6 constitutive equations). Specifying the boundary conditions, the boundary value problem is completely defined. To solve the system two approaches can be taken according to boundary conditions of the boundary value problem: a '''displacement formulation''', and a '''stress formulation'''. | |||
===Cylindrical coordinate form=== | |||
In cylindrical coordinates (<math>r,\theta,z</math>) the equations of motion are<ref name=Slau/> | |||
:<math> | |||
\begin{align} | |||
& \frac{\partial \sigma_{rr}}{\partial r} + \cfrac{1}{r}\frac{\partial \sigma_{r\theta}}{\partial \theta} + \frac{\partial \sigma_{rz}}{\partial z} + \cfrac{1}{r}(\sigma_{rr}-\sigma_{\theta\theta}) + F_r = \rho~\frac{\partial^2 u_r}{\partial t^2} \\ | |||
& \frac{\partial \sigma_{r\theta}}{\partial r} + \cfrac{1}{r}\frac{\partial \sigma_{\theta\theta}}{\partial \theta} + \frac{\partial \sigma_{\theta z}}{\partial z} + \cfrac{2}{r}\sigma_{r\theta} + F_\theta = \rho~\frac{\partial^2 u_\theta}{\partial t^2} \\ | |||
& \frac{\partial \sigma_{rz}}{\partial r} + \cfrac{1}{r}\frac{\partial \sigma_{\theta z}}{\partial \theta} + \frac{\partial \sigma_{zz}}{\partial z} + \cfrac{1}{r}\sigma_{rz} + F_z = \rho~\frac{\partial^2 u_z}{\partial t^2} | |||
\end{align} | |||
</math> | |||
The strain-displacement relations are | |||
:<math> | |||
\begin{align} | |||
\varepsilon_{rr} & = \cfrac{\partial u_r}{\partial r} ~;~~ | |||
\varepsilon_{\theta\theta} = \cfrac{1}{r}\left(\cfrac{\partial u_\theta}{\partial \theta} + u_r\right) ~;~~ | |||
\varepsilon_{zz} = \cfrac{\partial u_z}{\partial z} \\ | |||
\varepsilon_{r\theta} & = \cfrac{1}{2}\left(\cfrac{1}{r}\cfrac{\partial u_r}{\partial \theta} + \cfrac{\partial u_\theta}{\partial r}- \cfrac{u_\theta}{r}\right) ~;~~ | |||
\varepsilon_{\theta z} = \cfrac{1}{2}\left(\cfrac{\partial u_\theta}{\partial z} + \cfrac{1}{r}\cfrac{\partial u_z}{\partial \theta}\right) ~;~~ | |||
\varepsilon_{zr} = \cfrac{1}{2}\left(\cfrac{\partial u_r}{\partial z} + \cfrac{\partial u_z}{\partial r}\right) | |||
\end{align} | |||
</math> | |||
and the constitutive relations are the same as in Cartesian coordinates, except that the indices <math>1</math>,<math>2</math>,<math>3</math> now stand for <math>r</math>,<math>\theta</math>,<math>z</math>, respectively. | |||
===Spherical coordinate form === | |||
In spherical coordinates (<math>r,\theta,\phi</math>) the equations of motion are<ref name=Slau/> | |||
:<math> | |||
\begin{align} | |||
& \frac{\partial \sigma_{rr}}{\partial r} + \cfrac{1}{r}\frac{\partial \sigma_{r\theta}}{\partial \theta} + \cfrac{1}{r\sin\theta}\frac{\partial \sigma_{r\phi}}{\partial \phi} + \cfrac{1}{r}(2\sigma_{rr}-\sigma_{\theta\theta}-\sigma_{\phi\phi}+\sigma_{r\theta}\cot\theta) + F_r = \rho~\frac{\partial^2 u_r}{\partial t^2} \\ | |||
& \frac{\partial \sigma_{r\theta}}{\partial r} + \cfrac{1}{r}\frac{\partial \sigma_{\theta\theta}}{\partial \theta} + \cfrac{1}{r\sin\theta}\frac{\partial \sigma_{\theta \phi}}{\partial \phi} + \cfrac{1}{r}[(\sigma_{\theta\theta}-\sigma_{\phi\phi})\cot\theta + 3\sigma_{r\theta}] + F_\theta = \rho~\frac{\partial^2 u_\theta}{\partial t^2} \\ | |||
& \frac{\partial \sigma_{r\phi}}{\partial r} + \cfrac{1}{r}\frac{\partial \sigma_{\theta \phi}}{\partial \theta} + \cfrac{1}{r\sin\theta}\frac{\partial \sigma_{\phi\phi}}{\partial \phi} + \cfrac{1}{r}(2\sigma_{\theta\phi}\cot\theta+3\sigma_{r\phi}) + F_\phi = \rho~\frac{\partial^2 u_\phi}{\partial t^2} | |||
\end{align} | |||
</math> | |||
[[File:3D Spherical.svg|thumb|240px|right|Spherical coordinates (''r'', ''θ'', ''φ'') as commonly used in ''physics'': radial distance ''r'', polar angle ''θ'' ([[theta]]), and azimuthal angle ''φ'' ([[phi]]). The symbol ''ρ'' ([[rho]]) is often used instead of ''r''.]] | |||
The strain tensor in spherical coordinates is | |||
:<math> | |||
\begin{align} | |||
\varepsilon_{rr} & = \frac{\partial u_r}{\partial r}\\ | |||
\varepsilon_{\theta\theta}& = \frac{1}{r}\left(\frac{\partial u_\theta}{\partial \theta} + u_r\right)\\ | |||
\varepsilon_{\phi\phi} & = \frac{1}{r\sin\theta}\left(\frac{\partial u_\phi}{\partial \phi} + u_r\sin\theta + u_\theta\cos\theta\right)\\ | |||
\varepsilon_{r\theta} & = \frac{1}{2}\left(\frac{1}{r}\frac{\partial u_r}{\partial \theta} + \frac{\partial u_\theta}{\partial r}- \frac{u_\theta}{r}\right) \\ | |||
\varepsilon_{\theta \phi} & = \frac{1}{2r}\left[\frac{1}{\sin\theta}\frac{\partial u_\theta}{\partial \phi} +\left(\frac{\partial u_\phi}{\partial \theta}-u_\phi \cot\theta\right)\right]\\ | |||
\varepsilon_{r \phi} & = \frac{1}{2} \left(\frac{1}{r \sin \theta} \frac{\partial u_r}{\partial \phi} + \frac{\partial u_\phi}{\partial r} - \frac{u_\phi}{r}\right). | |||
\end{align} | |||
</math> | |||
== Isotropic homogeneous media == | |||
In [[Hooke's Law#Isotropic materials|isotropic]] media, the stiffness tensor gives the relationship between the stresses (resulting internal stresses) and the strains (resulting deformations). For an isotropic medium, the stiffness tensor has no preferred direction: an applied force will give the same displacements (relative to the direction of the force) no matter the direction in which the force is applied. In the isotropic case, the stiffness tensor may be written: | |||
:<math> C_{ijkl} | |||
= K \, \delta_{ij}\, \delta_{kl} | |||
+\mu\, (\delta_{ik}\delta_{jl}+\delta_{il}\delta_{jk}-\textstyle{\frac{2}{3}}\, \delta_{ij}\,\delta_{kl}) | |||
\,\!</math>{{citation needed|date=June 2012}} | |||
where <math>\delta_{ij}\,\!</math> is the [[Kronecker delta]], ''K'' is the [[bulk modulus]] (or incompressibility), and <math>\mu\,\!</math> is the [[shear modulus]] (or rigidity), two [[elastic moduli]]. If the medium is inhomogeneous, the isotropic model is sensible if either the medium is piecewise-constant or weakly inhomogeneous; in the strongly inhomogeneous smooth model, anisotropy has to be accounted for. If the medium is [[Homogeneous (chemistry)|homogeneous]], then the elastic moduli will be independent of the position in the medium. The constitutive equation may now be written as: | |||
:<math> \sigma_{ij} | |||
=K\delta_{ij}\varepsilon_{kk}+2\mu(\varepsilon_{ij}-\textstyle{\frac{1}{3}}\delta_{ij}\varepsilon_{kk}). | |||
\,\!</math> | |||
This expression separates the stress into a scalar part on the left which may be associated with a scalar pressure, and a traceless part on the right which may be associated with shear forces. A simpler expression is: | |||
:<math> \sigma_{ij} | |||
=\lambda \delta_{ij} \varepsilon_{kk}+2\mu\varepsilon_{ij} | |||
\,\!</math>{{citation needed|date=June 2012}} | |||
where λ is [[Lamé parameters|Lamé's first parameter]]. Since the constitutive equation is simply a set of linear equations, the strain may be expressed as a function of the stresses as:<ref name=sommerfeld>{{cite book |title= Mechanics of Deformable Bodies |last=Sommerfeld|first=Arnold |authorlink=Arnold Sommerfeld|year=1964 |publisher=Academic Press |location=New York}}</ref> | |||
:<math>\varepsilon_{ij} | |||
= \frac{1}{9K}\delta_{ij}\sigma_{kk} + \frac{1}{2\mu}\left(\sigma_{ij}-\textstyle{\frac{1}{3}}\delta_{ij}\sigma_{kk}\right) | |||
\,\!</math> | |||
which is again, a scalar part on the left and a traceless shear part on the right. More simply: | |||
:<math>\varepsilon_{ij} | |||
=\frac{1}{2\mu}\sigma_{ij}-\frac{\nu}{E}\delta_{ij}\sigma_{kk}=\frac{1}{E}[(1+\nu)\sigma_{ij}-\nu\delta_{ij}\sigma_{kk}] | |||
\,\!</math> | |||
where ν is [[Poisson's ratio]] and ''E'' is [[Young's modulus]]. | |||
===Elastostatics=== | |||
Elastostatics is the study of linear elasticity under the conditions of equilibrium, in which all forces on the elastic body sum to zero, and the displacements are not a function of time. The [[Momentum#Linear_momentum_for_a_system|equilibrium equations]] are then | |||
:<math> | |||
\sigma_{ji,j}+ F_i = 0. | |||
\,\!</math> | |||
:{| class="collapsible collapsed" width="30%" style="text-align:left" | |||
!Engineering notation | |||
|- | |||
|<math>\frac{\partial \sigma_x}{\partial x} + \frac{\partial \tau_{yx}}{\partial y} + \frac{\partial \tau_{zx}}{\partial z} + F_x = 0\,\!</math> | |||
<math>\frac{\partial \tau_{xy}}{\partial x} + \frac{\partial \sigma_y}{\partial y} + \frac{\partial \tau_{zy}}{\partial z} + F_y = 0\,\!</math> | |||
<math>\frac{\partial \tau_{xz}}{\partial x} + \frac{\partial \tau_{yz}}{\partial y} + \frac{\partial \sigma_z}{\partial z} + F_z = 0\,\!</math> | |||
|} | |||
This section will discuss only the isotropic homogeneous case. | |||
====Displacement formulation==== | |||
In this case, the displacements are prescribed everywhere in the boundary. In this approach, the strains and stresses are eliminated from the formulation, leaving the displacements as the unknowns to be solved for in the governing equations. | |||
First, the strain-displacement equations are substituted into the constitutive equations (Hooke's Law), eliminating the strains as unknowns: | |||
:<math>\begin{align} | |||
\sigma_{ij} &= \lambda \delta_{ij} \varepsilon_{kk}+2\mu\varepsilon_{ij} \\ | |||
&= \lambda\delta_{ij}u_{k,k}+\mu\left(u_{i,j}+u_{j,i}\right). \\ | |||
\end{align} | |||
\,\!</math> | |||
Differentiating yields: | |||
:<math> | |||
\sigma_{ij,j} = \lambda u_{k,ki}+\mu\left(u_{i,jj}+u_{j,ij}\right).\,\!</math> | |||
Substituting into the equilibrium equation yields: | |||
:<math>\lambda u_{k,ki}+\mu\left(u_{i,jj}+u_{j,ij}\right) +F_i=0\,\!</math> | |||
or | |||
:<math>\mu u_{i,jj}+(\mu+\lambda)u_{j,ij}+F_i=0\,\!</math> | |||
where <math>\lambda\,\!</math> and <math>\mu\,\!</math> are [[Lamé parameters]]. | |||
In this way, the only unknowns left are the displacements, hence the name for this formulation. The governing equations obtained in this manner are called '''Navier-Cauchy equations''' or, alternatively, the ''elastostatic equations''. | |||
:{| class="toccolours collapsible collapsed" width="60%" style="text-align:left" | |||
!Derivation of Navier-Cauchy equations in Engineering notation | |||
|- | |||
|First, the <math>x\,\!</math>-direction will be considered. Substituting the strain-displacement equations into the equilibrium equation in the <math>x\,\!</math>-direction we have | |||
:<math>\sigma_x = 2\mu\varepsilon_x + \lambda(\varepsilon_x + \varepsilon_y +\varepsilon_z)=2\mu\frac{\partial u_x}{\partial x}+\lambda\left(\frac{\partial u_x}{\partial x}+\frac{\partial u_y}{\partial y}+\frac{\partial u_z}{\partial z}\right)\,\!</math> | |||
:<math>\tau_{xy} = \mu\gamma_{xy}=\mu\left(\frac{\partial u_x}{\partial y}+\frac{\partial u_y}{\partial x}\right)\,\!</math> | |||
:<math>\tau_{xz} = \mu\gamma_{zx}=\mu\left(\frac{\partial u_z}{\partial x}+\frac{\partial u_x}{\partial z}\right)\,\!</math> | |||
Then substituting these equations into the equilibrium equation in the <math>x\,\!</math>-direction we have | |||
:<math>\frac{\partial \sigma_x}{\partial x} + \frac{\partial \tau_{yx}}{\partial y} + \frac{\partial \tau_{zx}}{\partial z} + F_x = 0\,\!</math> | |||
:<math>\frac{\partial}{\partial x}\left( 2\mu\frac{\partial u_x}{\partial x}+\lambda\left(\frac{\partial u_x}{\partial x}+\frac{\partial u_y}{\partial y}+\frac{\partial u_z}{\partial z}\right)\right) + \mu\frac{\partial}{\partial y}\left(\frac{\partial u_x}{\partial y}+\frac{\partial u_y}{\partial x}\right)+ \mu\frac{\partial}{\partial z}\left(\frac{\partial u_z}{\partial x}+\frac{\partial u_x}{\partial z}\right)+F_x=0\,\!</math> | |||
Using the assumption that <math>\mu</math> and <math>\lambda</math> are constant we can rearrange and get: | |||
:<math>\left(\lambda+\mu\right)\frac{\partial}{\partial x}\left(\frac{\partial u_x}{\partial x}+\frac{\partial u_y}{\partial y}+\frac{\partial u_z}{\partial z}\right)+\mu\left(\frac{\partial^2 u_x}{\partial x^2}+\frac{\partial^2 u_x}{\partial y^2}+\frac{\partial^2 u_x}{\partial z^2}\right)+F_x=0\,\!</math> | |||
Following the same procedure for the <math>y\,\!</math>-direction and <math>z\,\!</math>-direction we have | |||
:<math>\left(\lambda+\mu\right)\frac{\partial}{\partial y}\left(\frac{\partial u_x}{\partial x}+\frac{\partial u_y}{\partial y}+\frac{\partial u_z}{\partial z}\right)+\mu\left(\frac{\partial^2 u_y}{\partial x^2}+\frac{\partial^2 u_y}{\partial y^2}+\frac{\partial^2 u_y}{\partial z^2}\right)+F_y=0\,\!</math> | |||
:<math>\left(\lambda+\mu\right)\frac{\partial}{\partial z}\left(\frac{\partial u_x}{\partial x}+\frac{\partial u_y}{\partial y}+\frac{\partial u_z}{\partial z}\right)+\mu\left(\frac{\partial^2 u_z}{\partial x^2}+\frac{\partial^2 u_z}{\partial y^2}+\frac{\partial^2 u_z}{\partial z^2}\right)+F_z=0\,\!</math> | |||
These last 3 equations are the Navier-Cauchy equations, which can be also expressed in vector notation as | |||
:<math>(\lambda+\mu)\nabla(\nabla\cdot\mathbf{u})+\mu\nabla^2\mathbf{u}+\mathbf{F}=0\,\!</math> | |||
|} | |||
Once the displacement field has been calculated, the displacements can be replaced into the strain-displacement equations to solve for strains, which later are used in the constitutive equations to solve for stresses. | |||
===== The biharmonic equation ===== | |||
The elastostatic equation may be written: | |||
:<math>(\alpha^2-\beta^2)u_{j,ij}+ | |||
\beta^2u_{i,mm}=-F_i.\,\!</math> | |||
Taking the [[divergence]] of both sides of the elastostatic equation and assuming the body forces has zero divergence (homogeneous in domain) (<math>F_{i,i}=0\,\!</math>) we have | |||
:<math>(\alpha^2-\beta^2)u_{j,iij}+\beta^2u_{i,imm} = 0.\,\!</math> | |||
Noting that summed indices need not match, and that the partial derivatives commute, the two differential terms are seen to be the same and we have: | |||
:<math>\alpha^2u_{j,iij} = 0\,\!</math> | |||
from which we conclude that: | |||
:<math>u_{j,iij} = 0.\,\!</math> | |||
Taking the [[Laplacian]] of both sides of the elastostatic equation, and assuming in addition <math>F_{i,kk}=0\,\!</math>, we have | |||
:<math>(\alpha^2-\beta^2)u_{j,kkij}+\beta^2u_{i,kkmm}=0.\,\!</math> | |||
From the divergence equation, the first term on the left is zero (Note: again, the summed indices need not match) and we have: | |||
:<math>\beta^2u_{i,kkmm}=0\,\!</math> | |||
from which we conclude that: | |||
:<math>u_{i,kkmm}=0\,\!</math> | |||
or, in coordinate free notation <math>\nabla^4 \mathbf{u}=0\,\!</math> which is just the [[biharmonic equation]] in <math>\mathbf{u}\,\!</math>. | |||
====Stress formulation==== | |||
In this case, the surface tractions are prescribed everywhere on the surface boundary. In this approach, the strains and displacements are eliminated leaving the stresses as the unknowns to be solved for in the governing equations. Once the stress field is found, the strains are then found using the constitutive equations. | |||
There are six independent components of the stress tensor which need to be determined, yet in the displacement formulation, there are only three components of the displacement vector which need to be determined. This means that there are some constraints which must be placed upon the stress tensor, to reduce the number of degrees of freedom to three. Using the constitutive equations, these constraints are derived directly from corresponding constraints which must hold for the strain tensor, which also has six independent components. The constraints on the strain tensor are derivable directly from the definition of the strain tensor as a function of the displacement vector field, which means that these constraints introduce no new concepts or information. It is the constraints on the strain tensor that are most easily understood. If the elastic medium is visualized as a set of infinitesimal cubes in the unstrained state, then after the medium is strained, an arbitrary strain tensor must yield a situation in which the distorted cubes still fit together without overlapping. In other words, for a given strain, there must exist a continuous vector field (the displacement) from which that strain tensor can be derived. The constraints on the strain tensor that are required to assure that this is the case were discovered by Saint Venant, and are called the "[[Saint-Venant's compatibility condition|Saint Venant compatibility equations]]". These are 81 equations, 6 of which are independent non-trivial equations, which relate the different strain components. These are expressed in index notation as: | |||
:<math>\varepsilon_{ij,km}+\varepsilon_{km,ij}-\varepsilon_{ik,jm}-\varepsilon_{jm,ik}=0.\,\!</math> | |||
:{| class="collapsible collapsed" width="30%" style="text-align:left" | |||
!Engineering notation | |||
|- | |||
|<math>\frac{\partial^2 \epsilon_x}{\partial y^2} + \frac{\partial^2 \epsilon_y}{\partial x^2} = 2 \frac{\partial^2 \epsilon_{xy}}{\partial x \partial y}\,\!</math> | |||
<math>\frac{\partial^2 \epsilon_y}{\partial z^2} + \frac{\partial^2 \epsilon_z}{\partial y^2} = 2 \frac{\partial^2 \epsilon_{yz}}{\partial y \partial z}\,\!</math> | |||
<math>\frac{\partial^2 \epsilon_x}{\partial z^2} + \frac{\partial^2 \epsilon_z}{\partial x^2} = 2 \frac{\partial^2 \epsilon_{zx}}{\partial z \partial x}\,\!</math> | |||
<math>\frac{\partial^2 \epsilon_x}{\partial y \partial z} = \frac{\partial}{\partial x} \left ( -\frac{\partial \epsilon_{yz}}{\partial x} + \frac{\partial \epsilon_{zx}}{\partial y} + \frac{\partial \epsilon_{xy}}{\partial z}\right)\,\!</math> | |||
<math>\frac{\partial^2 \epsilon_y}{\partial z \partial x} = \frac{\partial}{\partial y} \left ( \frac{\partial \epsilon_{yz}}{\partial x} - \frac{\partial \epsilon_{zx}}{\partial y} + \frac{\partial \epsilon_{xy}}{\partial z}\right)\,\!</math> | |||
<math>\frac{\partial^2 \epsilon_z}{\partial x \partial y} = \frac{\partial}{\partial z} \left ( \frac{\partial \epsilon_{yz}}{\partial x} + \frac{\partial \epsilon_{zx}}{\partial y} - \frac{\partial \epsilon_{xy}}{\partial z}\right)\,\!</math> | |||
|} | |||
The strains in this equation are then expressed in terms of the stresses using the constitutive equations, which yields the corresponding constraints on the stress tensor. These constraints on the stress tensor are known as the ''Beltrami-Michell'' equations of compatibility: | |||
:<math>\sigma_{ij,kk}+\frac{1}{1+\nu}\sigma_{kk,ij}+F_{i,j}+F_{j,i}+\frac{\nu}{1-\nu}\delta_{i,j}F_{k,k}=0.\,\!</math> | |||
In the special situation where the body force is homogeneous, the above equations reduce to | |||
:<math> (1+\nu)\sigma_{ij,kk}+\sigma_{kk,ij}=0.\,\!</math> | |||
A necessary, but insufficient, condition for compatibility under this situation is <math>\boldsymbol{\nabla}^4\boldsymbol{\sigma} = \boldsymbol{0}</math> or <math>\sigma_{ij,kk\ell\ell} = 0</math>.<ref name=Slau/> | |||
These constraints, along with the equilibrium equation (or equation of motion for elastodynamics) allow the calculation of the stress tensor field. Once the stress field has been calculated from these equations, the strains can be obtained from the constitutive equations, and the displacement field from the strain-displacement equations. | |||
An alternative solution technique is to express the stress tensor in terms of [[stress functions]] which automatically yield a solution to the equilibrium equation. The stress functions then obey a single differential equation which corresponds to the compatibility equations. | |||
====Solutions for elastostatic cases==== | |||
:{| class="toccolours collapsible collapsed" width="60%" style="text-align:left" | |||
!Thomson's solution - point force in an infinite isotropic medium | |||
|- | |||
|The most important solution of the Navier-Cauchy or elastostatic equation is for that of a force acting at a point in an infinite isotropic medium. This solution was found by [[William Thomson, 1st Baron Kelvin|William Thomson]] (later Lord Kelvin) in 1848 (Thomson 1848). This solution is the analog of [[Coulomb's law]] in [[electrostatics]]. A derivation is given in Landau & Lifshitz.<ref name=LL>{{cite book |title=Theory of Elasticity |edition=3rd|last=Landau |first=L.D. |authorlink=Lev Landau |coauthors=[[Evgeny Lifshitz|Lifshitz, E. M.]] |year=1986 |publisher=Butterworth Heinemann |location=Oxford, England |isbn=0-7506-2633-X }}</ref>{{rp|§8}} Defining | |||
:<math>a=1-2\nu\,\!</math> | |||
:<math>b=2(1-\nu)=a+1\,\!</math> | |||
where <math>\nu\,\!</math> is Poisson's ratio, the solution may be expressed as | |||
:<math>u_i=G_{ik}F_k\,\!</math> | |||
where <math>F_k\,\!</math> is the force vector being applied at the point, and <math>G_{ik}\,\!</math> is a tensor [[Green's function]] which may be written in [[Cartesian coordinates]] as: | |||
:<math>G_{ik}= | |||
\frac{1}{4\pi\mu r}\left[ | |||
\left(1-\frac{1}{2b}\right)\delta_{ik}+\frac{1}{2b}\frac{x_i x_k}{r^2} | |||
\right] | |||
\,\!</math> | |||
It may be also compactly written as: | |||
:<math>G_{ik}= | |||
\frac{1}{4\pi\mu}\left[\frac{\delta_{ik}}{r}-\frac{1}{2b}\frac{\partial^2 r}{\partial x_i\partial x_k}\right] | |||
\,\!</math> | |||
and it may be explicitly written as: | |||
:<math>G_{ik}=\frac{1}{4\pi\mu r}\begin{bmatrix} | |||
1-\frac{1}{2b}+\frac{1}{2b}\frac{x^2}{r^2} & | |||
\frac{1}{2b}\frac{xy} {r^2} & | |||
\frac{1}{2b}\frac{xz} {r^2} \\ | |||
\frac{1}{2b}\frac{yx} {r^2} & | |||
1-\frac{1}{2b}+\frac{1}{2b}\frac{y^2}{r^2} & | |||
\frac{1}{2b}\frac{yz} {r^2} \\ | |||
\frac{1}{2b}\frac{zx} {r^2} & | |||
\frac{1}{2b}\frac{zy} {r^2} & | |||
1-\frac{1}{2b}+\frac{1}{2b}\frac{z^2}{r^2} | |||
\end{bmatrix} | |||
\,\!</math> | |||
In cylindrical coordinates (<math>\rho,\phi,z\,\!</math>) it may be written as: | |||
:<math>G_{ik}=\frac{1}{4\pi \mu r}\begin{bmatrix} | |||
1-\frac{1}{2b}\frac{z^2}{r^2}&0&\frac{1}{2b}\frac{\rho z}{r^2}\\ | |||
0&1-\frac{1}{2b}&0\\ | |||
\frac{1}{2b}\frac{z \rho}{r^2}&0&1-\frac{1}{2b}\frac{\rho^2}{r^2} | |||
\end{bmatrix} | |||
\,\!</math> | |||
where r is total distance to point. | |||
It is particularly helpful to write the displacement in cylindrical coordinates for a point force <math>F_z\,\!</math> directed along the z-axis. Defining <math>\hat{\mathbf{\rho}}\,\!</math> and <math>\hat{\mathbf{z}}\,\!</math> as unit vectors in the <math>\rho\,\!</math> and <math>z\,\!</math> directions respectively yields: | |||
:<math> | |||
\mathbf{u}=\frac{F_z}{4\pi\mu r}\left[\frac{1}{4(1-\nu)}\,\frac{\rho z}{r^2}\hat{\mathbf{\rho}} + \left(1-\frac{1}{4(1-\nu)}\,\frac{\rho^2}{r^2}\right)\hat{\mathbf{z}}\right] | |||
\,\!</math> | |||
It can be seen that there is a component of the displacement in the direction of the force, which diminishes, as is the case for the potential in electrostatics, as 1/r for large r. There is also an additional ρ-directed component. | |||
|} | |||
:{| class="toccolours collapsible collapsed" width="60%" style="text-align:left" | |||
!Boussinesq-Cerruti solution - point force at the origin of an infinite isotropic half-space | |||
|- | |||
|Another useful solution is that of a point force acting on the surface of an infinite half-space. It was derived by Boussinesq<ref>{{cite book |title= Application des potentiels à l'étude de l'équilibre et du mouvement des solides élastiques |last=Boussinesq|first=Joseph |authorlink=Joseph Boussinesq |year=1885 |publisher=Gauthier-Villars |location=Paris, France |url=http://name.umdl.umich.edu/ABV5032.0001.001 }}</ref> and a derivation is given in Landau & Lifshitz.<ref name=LL/>{{rp|§8}} In this case, the solution is again written as a Green's tensor which goes to zero at infinity, and the component of the stress tensor normal to the surface vanishes. This solution may be written in Cartesian coordinates as [note: a=(1-2ν) and b=2(1-ν), ν== Poissons ratio]: | |||
:<math>G_{ik}=\frac{1}{4\pi\mu}\begin{bmatrix} | |||
\frac{b}{r}+\frac{x^2}{r^3}-\frac{ax^2}{r(r+z)^2}-\frac{az}{r(r+z)} & | |||
\frac{xy}{r^3}-\frac{axy}{r(r+z)^2}& | |||
\frac{xz}{r^3}-\frac{ax}{r(r+z)}\\ | |||
\frac{yx}{r^3} -\frac{ayx}{r(r+z)^2}& | |||
\frac{b}{r}+\frac{y^2}{r^3}-\frac{ay^2}{r(r+z)^2}-\frac{az}{r(r+z)} & | |||
\frac{yz}{r^3} -\frac{ay}{r(r+z)}\\ | |||
\frac{zx}{r^3}+\frac{ax}{r(r+z)}& | |||
\frac{zy}{r^3}+\frac{ay}{r(r+z)}& | |||
\frac{b}{r}+\frac{z^2}{r^3} | |||
\end{bmatrix} | |||
\,\!</math> | |||
|} | |||
Other solutions: | |||
* Point force inside an infinite isotropic half-space<ref>{{cite journal |last=Mindlin |first= R. D.|authorlink=Raymond D. Mindlin |year=1936|title=Force at a point in the interior of a semi-infinite solid |journal=Physics |volume=7|issue= 5|pages=195–202 |id= |url= |quote=|doi=10.1063/1.1745385 |bibcode = 1936Physi...7..195M }}</ref> | |||
* Contact of two elastic bodies: the Hertz solution.<ref>{{cite journal |last=Hertz |first= Heinrich|authorlink=Heinrich Hertz |year=1882|title=Contact between solid elastic bodies |journal=Journ. Für reine und angewandte Math.|volume=92}}</ref> See also the page on [[Contact mechanics]]. | |||
=== Elastodynamics – the wave equation === | |||
{{Expand section|more principles, a brief explanation to each type of wave|discuss=Talk:Linear elasticity#New section needed|date=September 2010}} | |||
Elastodynamics is the study of '''elastic waves''' and involves linear elasticity with variation in time. An '''elastic wave''' is a type of [[mechanical wave]] that propagates in elastic or [[viscoelasticity|viscoelastic]] materials. The elasticity of the material provides the restoring [[force]] of the wave. When they occur in the [[Earth]] as the result of an [[earthquake]] or other disturbance, elastic waves are usually called [[seismic wave]]s. | |||
The '''[[wave equation]]''' of elastodynamics is simply the equilibrium equation of elastostatics with an additional inertial term: | |||
:<math> | |||
\sigma_{ji,j}+ F_i = \rho\,\ddot{u}_i = \rho\,\partial_{tt}u_i. | |||
\,\!</math> | |||
If the material is isotropic and homogeneous (i.e. the stiffness tensor is constant throughout the material), the '''elastodynamic wave equation''' has the form: | |||
:<math> | |||
\mu u_{i,jj}+(\mu+\lambda)u_{j,ij}+F_i=\rho\partial_{tt}u_i | |||
\quad \mathrm{or}\quad | |||
\mu\nabla^2\mathbf{u}+(\mu+\lambda)\nabla(\nabla\cdot\mathbf{u})+\mathbf{F}=\rho\frac{\partial^2\mathbf{u}}{\partial t^2}. | |||
\,\!</math> | |||
The elastodynamic wave equation can also be expressed as | |||
:<math> (\delta_{kl} \partial_{tt}-A_{kl}[\nabla])\, u_l | |||
= \frac{1}{\rho} F_k\,\!</math> | |||
where | |||
:<math> A_{kl}[\nabla]=\frac{1}{\rho} \, \partial_i \, C_{iklj} \, \partial_j\,\!</math> | |||
is the ''acoustic differential operator'', and <math> \delta_{kl}\,\!</math> is [[Kronecker delta]]. | |||
In [[Hooke's Law#Isotropic materials|isotropic]] media, the stiffness tensor has the form | |||
:<math> C_{ijkl} | |||
= K \, \delta_{ij}\, \delta_{kl} | |||
+\mu\, (\delta_{ik}\delta_{jl}+\delta_{il}\delta_{jk}-\frac{2}{3}\, \delta_{ij}\,\delta_{kl})\,\!</math> | |||
where | |||
<math>K\,\!</math> is the [[bulk modulus]] (or incompressibility), and | |||
<math>\mu\,\!</math> is the [[shear modulus]] (or rigidity), two [[elastic moduli]]. If the material is homogeneous (i.e. the stiffness tensor is constant throughout the material), the acoustic operator becomes: | |||
:<math>A_{ij}[\nabla]=\alpha^2 \partial_i\partial_j+\beta^2(\partial_m\partial_m\delta_{ij}-\partial_i\partial_j)\,\!</math> | |||
For [[plane waves]], the above differential operator becomes the ''acoustic algebraic operator'': | |||
:<math>A_{ij}[\mathbf{k}]=\alpha^2 k_ik_j+\beta^2(k_mk_m\delta_{ij}-k_ik_j)\,\!</math> | |||
where | |||
:<math> \alpha^2=\left(K+\frac{4}{3}\mu\right)/\rho \qquad \beta^2=\mu/\rho\,\!</math> | |||
are the [[eigenvalue]]s of <math>A[\hat{\mathbf{k}}]\,\!</math> with [[eigenvector]]s <math>\hat{\mathbf{u}}\,\!</math> parallel and orthogonal to the propagation direction <math>\hat{\mathbf{k}}\,\!</math>, respectively. In the seismological literature, the corresponding plane waves are called P-waves and S-waves (see [[Seismic wave]]). | |||
==Anisotropic homogeneous media == | |||
{{Main|Hooke's law}} | |||
For anisotropic media, the stiffness tensor <math> C_{ijkl}\,\!</math> is more complicated. The symmetry of the stress tensor <math>\sigma_{ij}\,\!</math> means that there are at most 6 different elements of stress. Similarly, there are at most 6 different elements of the strain tensor <math>\varepsilon_{ij}\,\!</math> . Hence the fourth-order stiffness tensor <math> C_{ijkl}\,\!</math> may be written as a matrix <math>C_{\alpha \beta}\,\!</math> (a tensor of second order). [[Voigt notation]] is the standard mapping for tensor indices, | |||
:<math> | |||
\begin{matrix} | |||
ij & =\\ | |||
\Downarrow & \\ | |||
\alpha & = | |||
\end{matrix} | |||
\begin{matrix} | |||
11 & 22 & 33 & 23,32 & 13,31 & 12,21 \\ | |||
\Downarrow & \Downarrow & \Downarrow & \Downarrow & \Downarrow & \Downarrow & \\ | |||
1 &2 & 3 & 4 & 5 & 6 | |||
\end{matrix}\,\!</math> | |||
With this notation, one can write the elasticity matrix for any linearly elastic medium as: | |||
:<math> C_{ijkl} \Rightarrow C_{\alpha \beta} =\begin{bmatrix} | |||
C_{11} & C_{12} & C_{13} & C_{14} & C_{15} & C_{16} \\ | |||
C_{12} & C_{22} & C_{23} & C_{24} & C_{25} & C_{26} \\ | |||
C_{13} & C_{23} & C_{33} & C_{34} & C_{35} & C_{36} \\ | |||
C_{14} & C_{24} & C_{34} & C_{44} & C_{45} & C_{46} \\ | |||
C_{15} & C_{25} & C_{35} & C_{45} & C_{55} & C_{56} \\ | |||
C_{16} & C_{26} & C_{36} & C_{46} & C_{56} & C_{66} | |||
\end{bmatrix}. | |||
\,\!</math> | |||
As shown, the matrix <math> C_{\alpha \beta}\,\!</math> is symmetric, this is a result of the existence of a strain energy density function which satisfies <math>\sigma_{ij}=\frac{\partial W}{\partial\varepsilon_{ij}}</math>. Hence, there are at most 21 different elements of <math> C_{\alpha \beta}\,\!</math>. | |||
The isotropic special case has 2 independent elements: | |||
:<math> C_{\alpha \beta} =\begin{bmatrix} | |||
K+4 \mu\ /3 & K-2 \mu\ /3 & K-2 \mu\ /3 & 0 & 0 & 0 \\ | |||
K-2 \mu\ /3 & K+4 \mu\ /3 & K-2 \mu\ /3 & 0 & 0 & 0 \\ | |||
K-2 \mu\ /3 & K-2 \mu\ /3 & K+4 \mu\ /3 & 0 & 0 & 0 \\ | |||
0 & 0 & 0 & \mu\ & 0 & 0 \\ | |||
0 & 0 & 0 & 0 & \mu\ & 0 \\ | |||
0 & 0 & 0 & 0 & 0 & \mu\ | |||
\end{bmatrix}. | |||
\,\!</math> | |||
The simplest anisotropic case, that of cubic symmetry has 3 independent elements: | |||
:<math> C_{\alpha \beta} =\begin{bmatrix} | |||
C_{11} & C_{12} & C_{12} & 0 & 0 & 0 \\ | |||
C_{12} & C_{11} & C_{12} & 0 & 0 & 0 \\ | |||
C_{12} & C_{12} & C_{11} & 0 & 0 & 0 \\ | |||
0 & 0 & 0 & C_{44} & 0 & 0 \\ | |||
0 & 0 & 0 & 0 & C_{44} & 0 \\ | |||
0 & 0 & 0 & 0 & 0 & C_{44} | |||
\end{bmatrix}. | |||
\,\!</math> | |||
The case of [[transverse isotropy]], also called polar anisotropy, (with a single axis (the 3-axis) of symmetry) has 5 independent elements: | |||
:<math> C_{\alpha \beta} =\begin{bmatrix} | |||
C_{11} & C_{11}-2C_{66} & C_{13} & 0 & 0 & 0 \\ | |||
C_{11}-2C_{66} & C_{11} & C_{13} & 0 & 0 & 0 \\ | |||
C_{13} & C_{13} & C_{33} & 0 & 0 & 0 \\ | |||
0 & 0 & 0 & C_{44} & 0 & 0 \\ | |||
0 & 0 & 0 & 0 & C_{44} & 0 \\ | |||
0 & 0 & 0 & 0 & 0 & C_{66} | |||
\end{bmatrix}. | |||
\,\!</math> | |||
When the transverse isotropy is weak (i.e. close to isotropy), an alternative parametrization utilizing [[Thomsen parameters]], is convenient for the formulas for wave speeds. | |||
The case of orthotropy (the symmetry of a brick) has 9 independent elements: | |||
:<math> C_{\alpha \beta} =\begin{bmatrix} | |||
C_{11} & C_{12} & C_{13} & 0 & 0 & 0 \\ | |||
C_{12} & C_{22} & C_{23} & 0 & 0 & 0 \\ | |||
C_{13} & C_{23} & C_{33} & 0 & 0 & 0 \\ | |||
0 & 0 & 0 & C_{44} & 0 & 0 \\ | |||
0 & 0 & 0 & 0 & C_{55} & 0 \\ | |||
0 & 0 & 0 & 0 & 0 & C_{66} | |||
\end{bmatrix}. | |||
\,\!</math> | |||
=== Elastodynamics === | |||
The elastodynamic wave equation for anisotropic media can be expressed as | |||
:<math> (\delta_{kl} \partial_{tt}-A_{kl}[\nabla])\, u_l | |||
= \frac{1}{\rho} F_k\,\!</math> | |||
where | |||
:<math> A_{kl}[\nabla]=\frac{1}{\rho} \, \partial_i \, C_{iklj} \, \partial_j\,\!</math> | |||
is the ''acoustic differential operator'', and <math> \delta_{kl}\,\!</math> is [[Kronecker delta]]. | |||
==== Plane waves and Christoffel equation ==== | |||
A ''plane wave'' has the form | |||
:<math> \mathbf{u}[\mathbf{x}, \, t] = U[\mathbf{k} \cdot \mathbf{x} - \omega \, t] \, \hat{\mathbf{u}}\,\!</math> | |||
with <math>\hat{\mathbf{u}}\,\!</math> of unit length. | |||
It is a solution of the wave equation with zero forcing, if and only if | |||
<math> \omega^2\,\!</math> and <math>\hat{\mathbf{u}}\,\!</math> constitute an eigenvalue/eigenvector pair of the | |||
''acoustic algebraic operator'' | |||
:<math> A_{kl}[\mathbf{k}]=\frac{1}{\rho} \, k_i \, C_{iklj} \, k_j.\,\!</math> | |||
This ''propagation condition'' (also known as the '''Christoffel equation''') may be written as | |||
:<math>A[\hat{\mathbf{k}}] \, \hat{\mathbf{u}}=c^2 \, \hat{\mathbf{u}}\,\!</math> | |||
where | |||
<math>\hat{\mathbf{k}} = \mathbf{k} / \sqrt{\mathbf{k}\cdot\mathbf{k}}\,\!</math> | |||
denotes propagation direction | |||
and <math>c=\omega/\sqrt{\mathbf{k}\cdot\mathbf{k}}\,\!</math> is phase velocity. | |||
==See also== | |||
{{Continuum mechanics|cTopic=[[Solid mechanics]]}} | |||
<div style="-moz-column-count:4; column-count:4;"> | |||
*[[Castigliano's method]] | |||
*[[Clapeyron's theorem (elasticity)]] | |||
*[[Contact mechanics]] | |||
*[[Deformation (mechanics)|Deformation]] | |||
*[[Elasticity (physics)]] | |||
*[[Hooke's law]] | |||
*[[Infinitesimal strain theory]] | |||
*[[Michell solution]] | |||
*[[Plasticity (physics)]] | |||
*[[Signorini problem]] | |||
*[[Spring system]] | |||
*[[Stress (mechanics)]] | |||
*[[Stress functions]] | |||
</div> | |||
== References == | |||
{{Reflist}} | |||
{{More footnotes|date=September 2010}} | |||
{{DEFAULTSORT:Linear Elasticity}} | |||
[[Category:Elasticity (physics)]] | |||
[[Category:Solid mechanics]] | |||
Revision as of 06:15, 27 December 2013
Linear elasticity is the mathematical study of how solid objects deform and become internally stressed due to prescribed loading conditions. Linear elasticity models materials as continua. Linear elasticity is a simplification of the more general nonlinear theory of elasticity and is a branch of continuum mechanics. The fundamental "linearizing" assumptions of linear elasticity are: infinitesimal strains or "small" deformations (or strains) and linear relationships between the components of stress and strain. In addition linear elasticity is valid only for stress states that do not produce yielding. These assumptions are reasonable for many engineering materials and engineering design scenarios. Linear elasticity is therefore used extensively in structural analysis and engineering design, often with the aid of finite element analysis.
Mathematical formulation
Equations governing a linear elastic boundary value problem are based on three tensor partial differential equations for the balance of linear momentum and six infinitesimal strain-displacement relations. The system of differential equations is completed by a set of linear algebraic constitutive relations.
Direct tensor form
In direct tensor form that is independent of the choice of coordinate system, these governing equations are:[1]
- Equation of motion, which is an expression of Newton's second law:
- Strain-displacement equations:
- Constitutive equations. For elastic materials, Hooke's law represents the material behavior and relates the unknown stresses and strains. The general equation for Hooke's law is
where is the Cauchy stress tensor, is the infinitesimal strain tensor, is the displacement vector, is the fourth-order stiffness tensor, is the body force per unit volume, is the mass density, represents the nabla operator and represents a transpose, represents the second derivative with respect to time, and is the inner product of two second-order tensors (summation over repeated indices is implied).
Cartesian coordinate form
Template:Einstein summation convention Expressed in terms of components with respect to a rectangular Cartesian coordinate system, the governing equations of linear elasticity are:[1]
- where the subscript is a shorthand for and indicates , is the Cauchy stress tensor, are the body forces, is the mass density, and is the displacement.
- These are 3 independent equations with 6 independent unknowns (stresses).
- Strain-displacement equations:
- where is the strain. These are 6 independent equations relating strains and displacements with 9 independent unknowns (strains and displacements).
- Constitutive equations. The equation for Hooke's law is:
- where is the stiffness tensor. These are 6 independent equations relating stresses and strains. The coefficients of the stiffness tensor can always be specified so that .
An elastostatic boundary value problem for an isotropic-homogeneous media is a system of 15 independent equations and equal number of unknowns (3 equilibrium equations, 6 strain-displacement equations, and 6 constitutive equations). Specifying the boundary conditions, the boundary value problem is completely defined. To solve the system two approaches can be taken according to boundary conditions of the boundary value problem: a displacement formulation, and a stress formulation.
Cylindrical coordinate form
In cylindrical coordinates () the equations of motion are[1]
The strain-displacement relations are
and the constitutive relations are the same as in Cartesian coordinates, except that the indices ,, now stand for ,,, respectively.
Spherical coordinate form
In spherical coordinates () the equations of motion are[1]
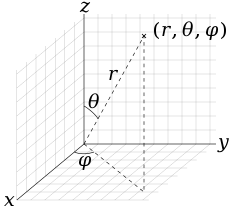
The strain tensor in spherical coordinates is
Isotropic homogeneous media
In isotropic media, the stiffness tensor gives the relationship between the stresses (resulting internal stresses) and the strains (resulting deformations). For an isotropic medium, the stiffness tensor has no preferred direction: an applied force will give the same displacements (relative to the direction of the force) no matter the direction in which the force is applied. In the isotropic case, the stiffness tensor may be written:
- Potter or Ceramic Artist Truman Bedell from Rexton, has interests which include ceramics, best property developers in singapore developers in singapore and scrabble. Was especially enthused after visiting Alejandro de Humboldt National Park.
where is the Kronecker delta, K is the bulk modulus (or incompressibility), and is the shear modulus (or rigidity), two elastic moduli. If the medium is inhomogeneous, the isotropic model is sensible if either the medium is piecewise-constant or weakly inhomogeneous; in the strongly inhomogeneous smooth model, anisotropy has to be accounted for. If the medium is homogeneous, then the elastic moduli will be independent of the position in the medium. The constitutive equation may now be written as:
This expression separates the stress into a scalar part on the left which may be associated with a scalar pressure, and a traceless part on the right which may be associated with shear forces. A simpler expression is:
- Potter or Ceramic Artist Truman Bedell from Rexton, has interests which include ceramics, best property developers in singapore developers in singapore and scrabble. Was especially enthused after visiting Alejandro de Humboldt National Park.
where λ is Lamé's first parameter. Since the constitutive equation is simply a set of linear equations, the strain may be expressed as a function of the stresses as:[2]
which is again, a scalar part on the left and a traceless shear part on the right. More simply:
where ν is Poisson's ratio and E is Young's modulus.
Elastostatics
Elastostatics is the study of linear elasticity under the conditions of equilibrium, in which all forces on the elastic body sum to zero, and the displacements are not a function of time. The equilibrium equations are then
This section will discuss only the isotropic homogeneous case.
Displacement formulation
In this case, the displacements are prescribed everywhere in the boundary. In this approach, the strains and stresses are eliminated from the formulation, leaving the displacements as the unknowns to be solved for in the governing equations. First, the strain-displacement equations are substituted into the constitutive equations (Hooke's Law), eliminating the strains as unknowns:
Differentiating yields:
Substituting into the equilibrium equation yields:
or
where and are Lamé parameters. In this way, the only unknowns left are the displacements, hence the name for this formulation. The governing equations obtained in this manner are called Navier-Cauchy equations or, alternatively, the elastostatic equations.
Once the displacement field has been calculated, the displacements can be replaced into the strain-displacement equations to solve for strains, which later are used in the constitutive equations to solve for stresses.
The biharmonic equation
The elastostatic equation may be written:
Taking the divergence of both sides of the elastostatic equation and assuming the body forces has zero divergence (homogeneous in domain) () we have
Noting that summed indices need not match, and that the partial derivatives commute, the two differential terms are seen to be the same and we have:
from which we conclude that:
Taking the Laplacian of both sides of the elastostatic equation, and assuming in addition , we have
From the divergence equation, the first term on the left is zero (Note: again, the summed indices need not match) and we have:
from which we conclude that:
or, in coordinate free notation which is just the biharmonic equation in .
Stress formulation
In this case, the surface tractions are prescribed everywhere on the surface boundary. In this approach, the strains and displacements are eliminated leaving the stresses as the unknowns to be solved for in the governing equations. Once the stress field is found, the strains are then found using the constitutive equations.
There are six independent components of the stress tensor which need to be determined, yet in the displacement formulation, there are only three components of the displacement vector which need to be determined. This means that there are some constraints which must be placed upon the stress tensor, to reduce the number of degrees of freedom to three. Using the constitutive equations, these constraints are derived directly from corresponding constraints which must hold for the strain tensor, which also has six independent components. The constraints on the strain tensor are derivable directly from the definition of the strain tensor as a function of the displacement vector field, which means that these constraints introduce no new concepts or information. It is the constraints on the strain tensor that are most easily understood. If the elastic medium is visualized as a set of infinitesimal cubes in the unstrained state, then after the medium is strained, an arbitrary strain tensor must yield a situation in which the distorted cubes still fit together without overlapping. In other words, for a given strain, there must exist a continuous vector field (the displacement) from which that strain tensor can be derived. The constraints on the strain tensor that are required to assure that this is the case were discovered by Saint Venant, and are called the "Saint Venant compatibility equations". These are 81 equations, 6 of which are independent non-trivial equations, which relate the different strain components. These are expressed in index notation as:
The strains in this equation are then expressed in terms of the stresses using the constitutive equations, which yields the corresponding constraints on the stress tensor. These constraints on the stress tensor are known as the Beltrami-Michell equations of compatibility:
In the special situation where the body force is homogeneous, the above equations reduce to
A necessary, but insufficient, condition for compatibility under this situation is or .[1]
These constraints, along with the equilibrium equation (or equation of motion for elastodynamics) allow the calculation of the stress tensor field. Once the stress field has been calculated from these equations, the strains can be obtained from the constitutive equations, and the displacement field from the strain-displacement equations.
An alternative solution technique is to express the stress tensor in terms of stress functions which automatically yield a solution to the equilibrium equation. The stress functions then obey a single differential equation which corresponds to the compatibility equations.
Solutions for elastostatic cases
Thomson's solution - point force in an infinite isotropic medium The most important solution of the Navier-Cauchy or elastostatic equation is for that of a force acting at a point in an infinite isotropic medium. This solution was found by William Thomson (later Lord Kelvin) in 1848 (Thomson 1848). This solution is the analog of Coulomb's law in electrostatics. A derivation is given in Landau & Lifshitz.[3]Primarily based on the most recent URA personal property value index (PPPI) flash estimates, we know that the PPPI, which represents the overall real property price development, has dipped in 2013Q4. That is the first dip the market has seen within the final 2 years.
To give you some perspective, the entire number of personal properties in Singapore (together with govt condominiums) is 297,689 in 2013Q3. Primarily based on the projection that there will be 19,302 units accomplished in 2014, the rise in residential models works out to be more than 6%. With a lot New Ec Launch Singapore provide, buyers might be spoilt for alternative and this in flip will lead to their reluctance to pay a premium for potential models. The complete textual content of the Copyright Act (Cap sixty three) and different statutes regarding IPR might be found on the Singapore Statutes Online Website online The Group's income jumped forty.1 p.c to $324.5 million from $231.6 million in FY 2013, lifted by increased development income and sales of growth properties in Singapore and China. Actual Estate Shopping for
One factor we've on this nation is a big group of "economists," and "market analysts." What's interesting about this group of real property market-watchers is that there are two very other ways wherein they predict Boomers will affect housing markets over the subsequent decade. Let's check out those two opposites and see how every can change the best way real property investors strategy their markets. The good news is that actual property buyers are prepared for either state of affairs, and there's profit in being ready. I'm excited and searching ahead to the alternatives both or each of these conditions will supply; thank you Boomers! Mapletree to further broaden past Asia Why fortune will favour the brave in Asia's closing real property frontier
The story of the 23.2 home begins with a stack of Douglas fir beams salvaged from varied demolished warehouses owned by the consumer's household for a number of generations. Design and structure innovator Omer Arbel, configured them to type a triangulated roof, which makes up one of the placing features of the home. The transient from the entrepreneur-proprietor was not solely to design a house that integrates antique wood beams, however one which erases the excellence between inside and exterior. Built on a gentle slope on a large rural acreage surrounded by two masses of previous-development forests, the indoors movement seamlessly to the outdoors and, from the within looking, one enjoys unobstructed views of the existing natural panorama which is preserved
First, there are typically extra rental transactions than gross sales transactions, to permit AV to be decided for each property primarily based on comparable properties. Second, movements in sale costs are more unstable than leases. Hence, utilizing rental transactions to derive the AV helps to maintain property tax more steady for property homeowners. If you're buying or trying to lease a property. It's tiring to call up individual property agent, organize appointments, coordinate timing and to go for particular person property viewing. What most individuals do is to have a property agent representing them who will arrange and coordinate the viewings for all the properties out there based mostly on your necessities & most well-liked timing. Rent Property District 12 Rent Property District thirteen
The Annual Worth of a property is mostly derived based mostly on the estimated annual hire that it may well fetch if it have been rented out. In determining the Annual Worth of a property, IRAS will think about the leases of similar properties within the vicinity, dimension and condition of the property, and different relevant components. The Annual Worth of a property is determined in the identical method regardless of whether the property is let-out, proprietor-occupied or vacant. The Annual Worth of land is determined at 5% of the market price of the land. When a constructing is demolished, the Annual Worth of the land is assessed by this method. Property Tax on Residential Properties Buyer Stamp Responsibility on Buy of Properties – Business and residential properties Rent House District 01
Within the event the Bank's valuation is decrease than the acquisition price, the purchaser has to pay the distinction between the purchase value and the Bank's valuation utilizing money. As such, the money required up-front might be increased so it's at all times essential to know the valuation of the property before making any offer. Appoint Lawyer The Bank will prepare for a proper valuation of the property by way of physical inspection The completion statement will present you the balance of the acquisition price that you must pay after deducting any deposit, pro-rated property tax and utility costs, upkeep prices, and different relevant expenses in addition to any fees payable to the agent and the lawyer. Stamp Responsibility Primarily based on the Purchase Price or Market Value, whichever is larger Definingwhere is Poisson's ratio, the solution may be expressed as
where is the force vector being applied at the point, and is a tensor Green's function which may be written in Cartesian coordinates as:
It may be also compactly written as:
and it may be explicitly written as:
In cylindrical coordinates () it may be written as:
where r is total distance to point.
It is particularly helpful to write the displacement in cylindrical coordinates for a point force directed along the z-axis. Defining and as unit vectors in the and directions respectively yields:
It can be seen that there is a component of the displacement in the direction of the force, which diminishes, as is the case for the potential in electrostatics, as 1/r for large r. There is also an additional ρ-directed component.
Boussinesq-Cerruti solution - point force at the origin of an infinite isotropic half-space Another useful solution is that of a point force acting on the surface of an infinite half-space. It was derived by Boussinesq[4] and a derivation is given in Landau & Lifshitz.[3]Primarily based on the most recent URA personal property value index (PPPI) flash estimates, we know that the PPPI, which represents the overall real property price development, has dipped in 2013Q4. That is the first dip the market has seen within the final 2 years.
To give you some perspective, the entire number of personal properties in Singapore (together with govt condominiums) is 297,689 in 2013Q3. Primarily based on the projection that there will be 19,302 units accomplished in 2014, the rise in residential models works out to be more than 6%. With a lot New Ec Launch Singapore provide, buyers might be spoilt for alternative and this in flip will lead to their reluctance to pay a premium for potential models. The complete textual content of the Copyright Act (Cap sixty three) and different statutes regarding IPR might be found on the Singapore Statutes Online Website online The Group's income jumped forty.1 p.c to $324.5 million from $231.6 million in FY 2013, lifted by increased development income and sales of growth properties in Singapore and China. Actual Estate Shopping for
One factor we've on this nation is a big group of "economists," and "market analysts." What's interesting about this group of real property market-watchers is that there are two very other ways wherein they predict Boomers will affect housing markets over the subsequent decade. Let's check out those two opposites and see how every can change the best way real property investors strategy their markets. The good news is that actual property buyers are prepared for either state of affairs, and there's profit in being ready. I'm excited and searching ahead to the alternatives both or each of these conditions will supply; thank you Boomers! Mapletree to further broaden past Asia Why fortune will favour the brave in Asia's closing real property frontier
The story of the 23.2 home begins with a stack of Douglas fir beams salvaged from varied demolished warehouses owned by the consumer's household for a number of generations. Design and structure innovator Omer Arbel, configured them to type a triangulated roof, which makes up one of the placing features of the home. The transient from the entrepreneur-proprietor was not solely to design a house that integrates antique wood beams, however one which erases the excellence between inside and exterior. Built on a gentle slope on a large rural acreage surrounded by two masses of previous-development forests, the indoors movement seamlessly to the outdoors and, from the within looking, one enjoys unobstructed views of the existing natural panorama which is preserved
First, there are typically extra rental transactions than gross sales transactions, to permit AV to be decided for each property primarily based on comparable properties. Second, movements in sale costs are more unstable than leases. Hence, utilizing rental transactions to derive the AV helps to maintain property tax more steady for property homeowners. If you're buying or trying to lease a property. It's tiring to call up individual property agent, organize appointments, coordinate timing and to go for particular person property viewing. What most individuals do is to have a property agent representing them who will arrange and coordinate the viewings for all the properties out there based mostly on your necessities & most well-liked timing. Rent Property District 12 Rent Property District thirteen
The Annual Worth of a property is mostly derived based mostly on the estimated annual hire that it may well fetch if it have been rented out. In determining the Annual Worth of a property, IRAS will think about the leases of similar properties within the vicinity, dimension and condition of the property, and different relevant components. The Annual Worth of a property is determined in the identical method regardless of whether the property is let-out, proprietor-occupied or vacant. The Annual Worth of land is determined at 5% of the market price of the land. When a constructing is demolished, the Annual Worth of the land is assessed by this method. Property Tax on Residential Properties Buyer Stamp Responsibility on Buy of Properties – Business and residential properties Rent House District 01
Within the event the Bank's valuation is decrease than the acquisition price, the purchaser has to pay the distinction between the purchase value and the Bank's valuation utilizing money. As such, the money required up-front might be increased so it's at all times essential to know the valuation of the property before making any offer. Appoint Lawyer The Bank will prepare for a proper valuation of the property by way of physical inspection The completion statement will present you the balance of the acquisition price that you must pay after deducting any deposit, pro-rated property tax and utility costs, upkeep prices, and different relevant expenses in addition to any fees payable to the agent and the lawyer. Stamp Responsibility Primarily based on the Purchase Price or Market Value, whichever is larger In this case, the solution is again written as a Green's tensor which goes to zero at infinity, and the component of the stress tensor normal to the surface vanishes. This solution may be written in Cartesian coordinates as [note: a=(1-2ν) and b=2(1-ν), ν== Poissons ratio]:
Other solutions:
- Point force inside an infinite isotropic half-space[5]
- Contact of two elastic bodies: the Hertz solution.[6] See also the page on Contact mechanics.
Elastodynamics – the wave equation
Template:Expand section Elastodynamics is the study of elastic waves and involves linear elasticity with variation in time. An elastic wave is a type of mechanical wave that propagates in elastic or viscoelastic materials. The elasticity of the material provides the restoring force of the wave. When they occur in the Earth as the result of an earthquake or other disturbance, elastic waves are usually called seismic waves.
The wave equation of elastodynamics is simply the equilibrium equation of elastostatics with an additional inertial term:
If the material is isotropic and homogeneous (i.e. the stiffness tensor is constant throughout the material), the elastodynamic wave equation has the form:
The elastodynamic wave equation can also be expressed as
where
is the acoustic differential operator, and is Kronecker delta.
In isotropic media, the stiffness tensor has the form
where is the bulk modulus (or incompressibility), and is the shear modulus (or rigidity), two elastic moduli. If the material is homogeneous (i.e. the stiffness tensor is constant throughout the material), the acoustic operator becomes:
For plane waves, the above differential operator becomes the acoustic algebraic operator:
where
are the eigenvalues of with eigenvectors parallel and orthogonal to the propagation direction , respectively. In the seismological literature, the corresponding plane waves are called P-waves and S-waves (see Seismic wave).
Anisotropic homogeneous media
Mining Engineer (Excluding Oil ) Truman from Alma, loves to spend time knotting, largest property developers in singapore developers in singapore and stamp collecting. Recently had a family visit to Urnes Stave Church.
For anisotropic media, the stiffness tensor is more complicated. The symmetry of the stress tensor means that there are at most 6 different elements of stress. Similarly, there are at most 6 different elements of the strain tensor . Hence the fourth-order stiffness tensor may be written as a matrix (a tensor of second order). Voigt notation is the standard mapping for tensor indices,
With this notation, one can write the elasticity matrix for any linearly elastic medium as:
As shown, the matrix is symmetric, this is a result of the existence of a strain energy density function which satisfies . Hence, there are at most 21 different elements of .
The isotropic special case has 2 independent elements:
The simplest anisotropic case, that of cubic symmetry has 3 independent elements:
The case of transverse isotropy, also called polar anisotropy, (with a single axis (the 3-axis) of symmetry) has 5 independent elements:
When the transverse isotropy is weak (i.e. close to isotropy), an alternative parametrization utilizing Thomsen parameters, is convenient for the formulas for wave speeds.
The case of orthotropy (the symmetry of a brick) has 9 independent elements:
Elastodynamics
The elastodynamic wave equation for anisotropic media can be expressed as
where
is the acoustic differential operator, and is Kronecker delta.
Plane waves and Christoffel equation
A plane wave has the form
with of unit length. It is a solution of the wave equation with zero forcing, if and only if and constitute an eigenvalue/eigenvector pair of the acoustic algebraic operator
This propagation condition (also known as the Christoffel equation) may be written as
where denotes propagation direction and is phase velocity.
See also
References
43 year old Petroleum Engineer Harry from Deep River, usually spends time with hobbies and interests like renting movies, property developers in singapore new condominium and vehicle racing. Constantly enjoys going to destinations like Camino Real de Tierra Adentro. Template:More footnotes
- ↑ 1.0 1.1 1.2 1.3 1.4 Slaughter, W. S., (2002), The linearized theory of elasticity, Birkhauser.
- ↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - ↑ 3.0 3.1 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - ↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - ↑ One of the biggest reasons investing in a Singapore new launch is an effective things is as a result of it is doable to be lent massive quantities of money at very low interest rates that you should utilize to purchase it. Then, if property values continue to go up, then you'll get a really high return on funding (ROI). Simply make sure you purchase one of the higher properties, reminiscent of the ones at Fernvale the Riverbank or any Singapore landed property Get Earnings by means of Renting
In its statement, the singapore property listing - website link, government claimed that the majority citizens buying their first residence won't be hurt by the new measures. Some concessions can even be prolonged to chose teams of consumers, similar to married couples with a minimum of one Singaporean partner who are purchasing their second property so long as they intend to promote their first residential property. Lower the LTV limit on housing loans granted by monetary establishments regulated by MAS from 70% to 60% for property purchasers who are individuals with a number of outstanding housing loans on the time of the brand new housing purchase. Singapore Property Measures - 30 August 2010 The most popular seek for the number of bedrooms in Singapore is 4, followed by 2 and three. Lush Acres EC @ Sengkang
Discover out more about real estate funding in the area, together with info on international funding incentives and property possession. Many Singaporeans have been investing in property across the causeway in recent years, attracted by comparatively low prices. However, those who need to exit their investments quickly are likely to face significant challenges when trying to sell their property – and could finally be stuck with a property they can't sell. Career improvement programmes, in-house valuation, auctions and administrative help, venture advertising and marketing, skilled talks and traisning are continuously planned for the sales associates to help them obtain better outcomes for his or her shoppers while at Knight Frank Singapore. No change Present Rules
Extending the tax exemption would help. The exemption, which may be as a lot as $2 million per family, covers individuals who negotiate a principal reduction on their existing mortgage, sell their house short (i.e., for lower than the excellent loans), or take part in a foreclosure course of. An extension of theexemption would seem like a common-sense means to assist stabilize the housing market, but the political turmoil around the fiscal-cliff negotiations means widespread sense could not win out. Home Minority Chief Nancy Pelosi (D-Calif.) believes that the mortgage relief provision will be on the table during the grand-cut price talks, in response to communications director Nadeam Elshami. Buying or promoting of blue mild bulbs is unlawful.
A vendor's stamp duty has been launched on industrial property for the primary time, at rates ranging from 5 per cent to 15 per cent. The Authorities might be trying to reassure the market that they aren't in opposition to foreigners and PRs investing in Singapore's property market. They imposed these measures because of extenuating components available in the market." The sale of new dual-key EC models will even be restricted to multi-generational households only. The models have two separate entrances, permitting grandparents, for example, to dwell separately. The vendor's stamp obligation takes effect right this moment and applies to industrial property and plots which might be offered inside three years of the date of buy. JLL named Best Performing Property Brand for second year running
The data offered is for normal info purposes only and isn't supposed to be personalised investment or monetary advice. Motley Fool Singapore contributor Stanley Lim would not personal shares in any corporations talked about. Singapore private home costs increased by 1.eight% within the fourth quarter of 2012, up from 0.6% within the earlier quarter. Resale prices of government-built HDB residences which are usually bought by Singaporeans, elevated by 2.5%, quarter on quarter, the quickest acquire in five quarters. And industrial property, prices are actually double the levels of three years ago. No withholding tax in the event you sell your property. All your local information regarding vital HDB policies, condominium launches, land growth, commercial property and more
There are various methods to go about discovering the precise property. Some local newspapers (together with the Straits Instances ) have categorised property sections and many local property brokers have websites. Now there are some specifics to consider when buying a 'new launch' rental. Intended use of the unit Every sale begins with 10 p.c low cost for finish of season sale; changes to 20 % discount storewide; follows by additional reduction of fiftyand ends with last discount of 70 % or extra. Typically there is even a warehouse sale or transferring out sale with huge mark-down of costs for stock clearance. Deborah Regulation from Expat Realtor shares her property market update, plus prime rental residences and houses at the moment available to lease Esparina EC @ Sengkang - ↑ One of the biggest reasons investing in a Singapore new launch is an effective things is as a result of it is doable to be lent massive quantities of money at very low interest rates that you should utilize to purchase it. Then, if property values continue to go up, then you'll get a really high return on funding (ROI). Simply make sure you purchase one of the higher properties, reminiscent of the ones at Fernvale the Riverbank or any Singapore landed property Get Earnings by means of Renting
In its statement, the singapore property listing - website link, government claimed that the majority citizens buying their first residence won't be hurt by the new measures. Some concessions can even be prolonged to chose teams of consumers, similar to married couples with a minimum of one Singaporean partner who are purchasing their second property so long as they intend to promote their first residential property. Lower the LTV limit on housing loans granted by monetary establishments regulated by MAS from 70% to 60% for property purchasers who are individuals with a number of outstanding housing loans on the time of the brand new housing purchase. Singapore Property Measures - 30 August 2010 The most popular seek for the number of bedrooms in Singapore is 4, followed by 2 and three. Lush Acres EC @ Sengkang
Discover out more about real estate funding in the area, together with info on international funding incentives and property possession. Many Singaporeans have been investing in property across the causeway in recent years, attracted by comparatively low prices. However, those who need to exit their investments quickly are likely to face significant challenges when trying to sell their property – and could finally be stuck with a property they can't sell. Career improvement programmes, in-house valuation, auctions and administrative help, venture advertising and marketing, skilled talks and traisning are continuously planned for the sales associates to help them obtain better outcomes for his or her shoppers while at Knight Frank Singapore. No change Present Rules
Extending the tax exemption would help. The exemption, which may be as a lot as $2 million per family, covers individuals who negotiate a principal reduction on their existing mortgage, sell their house short (i.e., for lower than the excellent loans), or take part in a foreclosure course of. An extension of theexemption would seem like a common-sense means to assist stabilize the housing market, but the political turmoil around the fiscal-cliff negotiations means widespread sense could not win out. Home Minority Chief Nancy Pelosi (D-Calif.) believes that the mortgage relief provision will be on the table during the grand-cut price talks, in response to communications director Nadeam Elshami. Buying or promoting of blue mild bulbs is unlawful.
A vendor's stamp duty has been launched on industrial property for the primary time, at rates ranging from 5 per cent to 15 per cent. The Authorities might be trying to reassure the market that they aren't in opposition to foreigners and PRs investing in Singapore's property market. They imposed these measures because of extenuating components available in the market." The sale of new dual-key EC models will even be restricted to multi-generational households only. The models have two separate entrances, permitting grandparents, for example, to dwell separately. The vendor's stamp obligation takes effect right this moment and applies to industrial property and plots which might be offered inside three years of the date of buy. JLL named Best Performing Property Brand for second year running
The data offered is for normal info purposes only and isn't supposed to be personalised investment or monetary advice. Motley Fool Singapore contributor Stanley Lim would not personal shares in any corporations talked about. Singapore private home costs increased by 1.eight% within the fourth quarter of 2012, up from 0.6% within the earlier quarter. Resale prices of government-built HDB residences which are usually bought by Singaporeans, elevated by 2.5%, quarter on quarter, the quickest acquire in five quarters. And industrial property, prices are actually double the levels of three years ago. No withholding tax in the event you sell your property. All your local information regarding vital HDB policies, condominium launches, land growth, commercial property and more
There are various methods to go about discovering the precise property. Some local newspapers (together with the Straits Instances ) have categorised property sections and many local property brokers have websites. Now there are some specifics to consider when buying a 'new launch' rental. Intended use of the unit Every sale begins with 10 p.c low cost for finish of season sale; changes to 20 % discount storewide; follows by additional reduction of fiftyand ends with last discount of 70 % or extra. Typically there is even a warehouse sale or transferring out sale with huge mark-down of costs for stock clearance. Deborah Regulation from Expat Realtor shares her property market update, plus prime rental residences and houses at the moment available to lease Esparina EC @ Sengkang

![{\displaystyle {\boldsymbol {\varepsilon }}={\tfrac {1}{2}}\left[{\boldsymbol {\nabla }}\mathbf {u} +({\boldsymbol {\nabla }}\mathbf {u} )^{T}\right]\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a6e0607243656ca3e21e8a5080c860cb6b599e57)












































![{\displaystyle {\begin{aligned}&{\frac {\partial \sigma _{rr}}{\partial r}}+{\cfrac {1}{r}}{\frac {\partial \sigma _{r\theta }}{\partial \theta }}+{\cfrac {1}{r\sin \theta }}{\frac {\partial \sigma _{r\phi }}{\partial \phi }}+{\cfrac {1}{r}}(2\sigma _{rr}-\sigma _{\theta \theta }-\sigma _{\phi \phi }+\sigma _{r\theta }\cot \theta )+F_{r}=\rho ~{\frac {\partial ^{2}u_{r}}{\partial t^{2}}}\\&{\frac {\partial \sigma _{r\theta }}{\partial r}}+{\cfrac {1}{r}}{\frac {\partial \sigma _{\theta \theta }}{\partial \theta }}+{\cfrac {1}{r\sin \theta }}{\frac {\partial \sigma _{\theta \phi }}{\partial \phi }}+{\cfrac {1}{r}}[(\sigma _{\theta \theta }-\sigma _{\phi \phi })\cot \theta +3\sigma _{r\theta }]+F_{\theta }=\rho ~{\frac {\partial ^{2}u_{\theta }}{\partial t^{2}}}\\&{\frac {\partial \sigma _{r\phi }}{\partial r}}+{\cfrac {1}{r}}{\frac {\partial \sigma _{\theta \phi }}{\partial \theta }}+{\cfrac {1}{r\sin \theta }}{\frac {\partial \sigma _{\phi \phi }}{\partial \phi }}+{\cfrac {1}{r}}(2\sigma _{\theta \phi }\cot \theta +3\sigma _{r\phi })+F_{\phi }=\rho ~{\frac {\partial ^{2}u_{\phi }}{\partial t^{2}}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/beeff6e05d12be13c23e0e9934f192af0cdfd89d)
![{\displaystyle {\begin{aligned}\varepsilon _{rr}&={\frac {\partial u_{r}}{\partial r}}\\\varepsilon _{\theta \theta }&={\frac {1}{r}}\left({\frac {\partial u_{\theta }}{\partial \theta }}+u_{r}\right)\\\varepsilon _{\phi \phi }&={\frac {1}{r\sin \theta }}\left({\frac {\partial u_{\phi }}{\partial \phi }}+u_{r}\sin \theta +u_{\theta }\cos \theta \right)\\\varepsilon _{r\theta }&={\frac {1}{2}}\left({\frac {1}{r}}{\frac {\partial u_{r}}{\partial \theta }}+{\frac {\partial u_{\theta }}{\partial r}}-{\frac {u_{\theta }}{r}}\right)\\\varepsilon _{\theta \phi }&={\frac {1}{2r}}\left[{\frac {1}{\sin \theta }}{\frac {\partial u_{\theta }}{\partial \phi }}+\left({\frac {\partial u_{\phi }}{\partial \theta }}-u_{\phi }\cot \theta \right)\right]\\\varepsilon _{r\phi }&={\frac {1}{2}}\left({\frac {1}{r\sin \theta }}{\frac {\partial u_{r}}{\partial \phi }}+{\frac {\partial u_{\phi }}{\partial r}}-{\frac {u_{\phi }}{r}}\right).\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/aaa90ca80dd65296f4517746b485f85f251972d8)






![{\displaystyle \varepsilon _{ij}={\frac {1}{2\mu }}\sigma _{ij}-{\frac {\nu }{E}}\delta _{ij}\sigma _{kk}={\frac {1}{E}}[(1+\nu )\sigma _{ij}-\nu \delta _{ij}\sigma _{kk}]\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d536edfc67991ed5285f128282844577ff5196ab)


















































![{\displaystyle G_{ik}={\frac {1}{4\pi \mu r}}\left[\left(1-{\frac {1}{2b}}\right)\delta _{ik}+{\frac {1}{2b}}{\frac {x_{i}x_{k}}{r^{2}}}\right]\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4ae6eec372f36fedae643a7970673650ddf8c071)
![{\displaystyle G_{ik}={\frac {1}{4\pi \mu }}\left[{\frac {\delta _{ik}}{r}}-{\frac {1}{2b}}{\frac {\partial ^{2}r}{\partial x_{i}\partial x_{k}}}\right]\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/efd7ca63a668d8e1973bbcb2d0a492801e16d8b1)






![{\displaystyle \mathbf {u} ={\frac {F_{z}}{4\pi \mu r}}\left[{\frac {1}{4(1-\nu )}}\,{\frac {\rho z}{r^{2}}}{\hat {\mathbf {\rho } }}+\left(1-{\frac {1}{4(1-\nu )}}\,{\frac {\rho ^{2}}{r^{2}}}\right){\hat {\mathbf {z} }}\right]\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ca2688a666f8e3a5077f35ea555c5f40c3c02d3a)



![{\displaystyle (\delta _{kl}\partial _{tt}-A_{kl}[\nabla ])\,u_{l}={\frac {1}{\rho }}F_{k}\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1170a6d4fc50db1e67d7ece571f8815ed0d82acd)
![{\displaystyle A_{kl}[\nabla ]={\frac {1}{\rho }}\,\partial _{i}\,C_{iklj}\,\partial _{j}\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4e6ab1a06de454511eb01aaafe2f3a47f95ed75f)



![{\displaystyle A_{ij}[\nabla ]=\alpha ^{2}\partial _{i}\partial _{j}+\beta ^{2}(\partial _{m}\partial _{m}\delta _{ij}-\partial _{i}\partial _{j})\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2065c43b609ab33ad188b4523c5e158c083037aa)
![{\displaystyle A_{ij}[\mathbf {k} ]=\alpha ^{2}k_{i}k_{j}+\beta ^{2}(k_{m}k_{m}\delta _{ij}-k_{i}k_{j})\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/102975b7b7b0ada9c6a67d4dbfd7492a00ac0749)

![{\displaystyle A[{\hat {\mathbf {k} }}]\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/694e068b9f7b017a7ed7ba55d58e44a00720c27e)













![{\displaystyle \mathbf {u} [\mathbf {x} ,\,t]=U[\mathbf {k} \cdot \mathbf {x} -\omega \,t]\,{\hat {\mathbf {u} }}\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f5fb9c0fd19c9542bc3b7b8bcb8bb4064cc57303)

![{\displaystyle A_{kl}[\mathbf {k} ]={\frac {1}{\rho }}\,k_{i}\,C_{iklj}\,k_{j}.\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c3ed36d0ce72053d663fe22aa1905ada7e291353)
![{\displaystyle A[{\hat {\mathbf {k} }}]\,{\hat {\mathbf {u} }}=c^{2}\,{\hat {\mathbf {u} }}\,\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/26b43318e8405f0efc4e62587fa955bcc5d00c10)

